Tools of the Cold-Atom Trade: Evaporative Cooling

In our last installment of the cold-atom toolbox series, we talked about why you need magnetic traps to get to really ultra-cold samples-- because the light scattering involved in laser cooling limits you to a temperature that's too high for making Bose-Einstein condensation (BEC). This time out, we'll talk about how you actually get to those ultra-cold temperatures.

What do you mean? I assumed it was just part of the trapping process? No, because the forces involved in magnetic trapping are like those involved in optical dipole traps. In physics jargon, they're "conservative" forces, which can't produce cooling-- a sample of atoms in the trap will just move back and forth with constant total energy. To get cooling, you need a dissipative force, like the absorption and emission of photons in laser cooling.

But I thought you said you couldn't use photon scattering to get to BEC? Right, so you need to find another way to introduce dissipation into the system. Without using laser light to do it, because of the heating induced by photon scattering.

In the end, it turns out that there's really only one thing you can do: you can throw atoms out of the trap.

Yeah, but how does that help you? Just removing atoms at random doesn't help, but if you can selectively remove the fast-moving atoms, you can cool your sample.

Wait, fast-moving atoms? I thought these were all cold atoms? Well, fast is a relative term. The important thing here is that, as we discussed a while back regarding that "negative temperature" paper the temperature is a property of a distribution of atoms. The temeprature is simply related to the average kinetic energy of the sample, but the atoms don't all have the average energy-- instead, they're distributed over a range of energies about that average.

This means that no matter how cold the temperature of the sample is, there will always be some atoms with energies above the average energy. And if you can selectively remove those atoms, they take away a greater-than average amount of energy from the sample as a whole, meaning that the average energy of the remaining atoms is necessarily lower.

Yeah, but how is this possible? Isn't removing the fast-moving atoms a Maxwell's Demon sort of problem? I thought that was impossible. It's not impossible to set up a Maxwell Demon, just highly improbable. There's nothing in principle wrong with the idea-- what's impossible to do with a demon is to reduce the entropy of a gas without producing a corresponding increase in the entropy of the rest of the universe. If you work it all out, it turns out that the action of the "demon" will necessarily produce an increase in entropy that matches or exceeds the decrease in entropy of sorting the atoms by speed, so everything is okay.

But that's unnecessarily complicated-- it turns out that you don't need quasi-omniscient anthropomorphic personifications to make this work, just a clever use of your magnetic trap. Specifically, the fact that your trapped atoms are in one particular internal state, and there are other states out there that are not trapped.

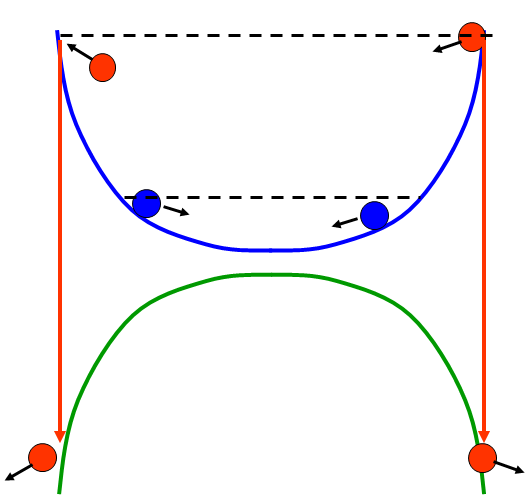
How does that help? Well, the cartoon version looks like this:

Cartoon version of evaporative cooling in a magnetic trap.

The blue and green lines represent the internal energy of an atom in the trapped and untrapped states, respectively. Initially, all the atoms are in the trapped state, which has a shape resembling a sort of bowl (in reality, it's usually a parabola, but I drew this quickly and didn't make the shape all that accurate). The atoms in the sample move around in this bowl, basically "sloshing" back and forth, turning around when their total energy matches the internal energy (because once all the energy available to the atom has gone into internal energy, it has to have zero kinetic energy-- that is, come to a stop for an instant).

The key realization is that the "hot" atoms (red circles) reach their turning points farther out from the center of the trap than the "cold" atoms (blue circles). Which means if you want to selectively remove "hot" atoms, you just need to remove those atoms that reach the outer edge of the trap.

Yeah, but how do you do that? That's where the untrapped states come in. If you do something to flip the atom from the trapped state to the untrapped state, it will feel a force pushing it away from the center-- the internal energy looks like the green curve, rather than the blue, and it can reduce that energy by moving away. And you can selectively change the state by using radio-frequency light.

I thought you said you weren't allowed to have any light? I said you couldn't have laser light. The RF photons used for evaporation are so low-energy that they don't change the momentum of the atoms significantly. And anyway, the only atoms interacting with them get removed from the trap.

But how do you select the "hot" atoms to remove? By the frequency of the light. See, to drive an atom from one state to the other, you need a frequency that matches the energy difference between the two states. That difference depends on the magnetic field, which depends on the position. Out near the edge of the trap, the energy difference is big, so you need a high frequency to flip the state, but closer to the center the energy difference is small, and the frequency is lower. You can basically dial in the position at which you remove atoms, and thus the energy of the atoms being removed, by choosing the frequency of RF that you apply.

That sounds... almost too good to be true. It's a little trickier than that description makes it sound, but it works very well. People in the field refer to it as an "RF knife," as if you're shaving away the atoms at the outer edge of the cloud. And if you use a TOP trap, you get an extra bonus bit of evaporation from the zero-field point, which orbits the center of the trap in a circle of some radius. Atoms crossing that "circle of death" can flip their state to the untrapped state as well, and again, this selectively removes atoms in the outer part of the trap, taking away the hot atoms.

But once you've removed the hot atoms, aren't you done cooling? I mean, they're gone, so what do you do next? Well, there are a couple of things that happen at that point. One is that the atoms collide with each other, and "re-thermalize." The total energy of the sample decreases because you've taken the hot atoms out, leaving a sort of truncated thermal distribution. collisions between atoms will redistribute this energy a little bit-- sometimes when two low-energy atoms collide, one will end up with higher energy, while the other moves to lower energy-- and pretty soon, you have a thermal distribution matching Maxwell-Boltzmann distribution again.

Then you can remove the highest-energy atoms from that, by reducing the frequency of the RF knife a little bit. That moves the point where you're taking atoms out in, and lowers the energy of the atoms you're removing. But they're still the hottest atoms in the trap, so the temperature decreases. And you re-thermalize again, and move the frequency again, and so on.

That's pretty slick. Yeah, it is. It's trickier than that description sounds, of course-- the thermalization depends on the collisional properties of the atoms, and there are both "good" collisions that redistribute energy and "bad" collisions that randomly remove atoms from the trap, so you have to make sure there are more good collisions than bad. But in the end, this process gets you lower temperature. And, incidentally, an increase in density, which you also need for BEC. So it's a great method all the way around.

Wait, how does it increase the density? The colder atoms left behind take up less room in the trap, because they have their turning points closer to the center. Meaning they're packed into a smaller volume, and thus have a higher density.

This still sounds too good to be true. Are you sure this is legal? It absolutely works, I swear to you. This is the means by which dozens of groups all over the world produce BEC, probably hundreds or thousands of times in a typical day.

But you don't need exotic physics experiments to prove that this process is physically allowed. Just go get a cup of coffee or tea, and don't drink it.

Don't drink it? Right. If you don't drink it, but just let it sit there, some time later, you'll come back to cold coffee or tea. This happens in part because of a process very similar to what cold-atom physicists do to make BEC: the "hottest" molecules in the hot coffee evaporate away as steam, and the molecules left behind have lower energy, and thus a colder temperature. That gives the process its name, "evaporative cooling."

But, but... Entropy? To be honest, I've never thought about the thermodynamics of this in all that much detail; since it undeniably works to make BEC, I feel safe in assuming that the entropy of the universe has increased as a result of this process, even as the condensate itself forms a low-entropy state. The decrease in the entropy of the atoms that go into the condensate is matched by an increase in the much greater number of atoms that left the trap, plus the RF photons and whatever else.

OK, I guess. But that brings up another point: doesn't this involve throwing a whole bunch of atoms away? Absolutely. The initial MOT that people load into a magnetic trap for evaporative cooling will usually have something like a billion or ten billion atoms. A million-atom BEC is a pretty healthy size, so you're throwing away around 99.9% of the atoms you start with in order to get the final BEC.

But that's the price you have to pay to get to those temperatures. And a million atoms is still plenty to work with to study the properties of BEC and the other cool quantum effects that it lets you explore.

I guess so. But, hey, you also alluded to making BEC in optical traps. How does that work? The basic principle is the same-- they do evaporative cooling. They generally don't use RF evaporation, though, because the size of the traps and the energy level shifts are different. Instead, they just provide a way for the trapped atoms to "leak" out of the trap, provided they have high enough energy. Then they can reduce the laser intensity, lowering the energy limit, evaporating hot atoms just like in a magnetic trap.

Optical traps tend to be smaller, so the collision rate is higher, and when you work it all out, the evaporation process proceeds faster, but the idea of the process is essentially the same: throw out hot atoms, keep cold ones.

If you want to play around with it, they have a cool little game at the Physics 2000 project at the University of Colorado. It requires Java, though, which might almost be more hassle than it's worth for the fun of evaporatively cooling simulated atoms.

So, how do you know when you've made a BEC? Do you stick in a thermometer, or something? No, you just take a picture of the atoms. These then get turned into three-dimensional plots of the density profile, like the famous shot that Cornell and Wieman used to announce they had gotten BEC, which is the "featured image" at the top of the post. The making and interpretation of those images is a critical part of cold-atom physics, but we'll save that for another post. Any other questions before then?

Just one: Can I drink this coffee now, before it evaporates away to BEC? Sure, go right ahead.