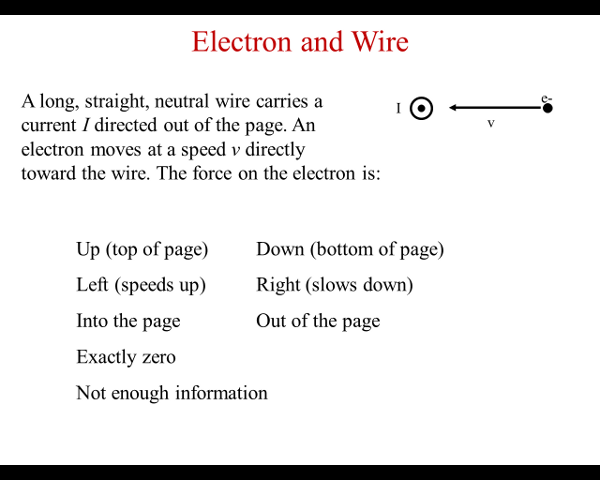
Every now and then, I run across a question in class that I genuinely don't know how to answer. If I'm lucky, this happens when I'm prepping a class, rather than when a student asks it live. Like today, when I noticed the above discussion question in my slides (reproduced at the bottom as well for those reading via RSS).
The discussion question itself is perfectly straightforward-- the current in the wire creates a magnetic field, the moving electron interacts with that, and getting the direction of the force is a straightforward right-hand-rule problem. I'll pose this to the class and ask them to work out their answers on small white boards, then "poll" the class by having them hold up the boards.
The return question, though, that I don't entirely expect somebody to ask, but realized I don't know the answer to, is what happens to the wire? Most of the time, when I do this kind of one-object-interacting-with-another question, I like to look at both action and reaction forces. But here, I don't think that works-- the magnetic field of the electron is zero straight ahead of it, and in opposite directions on either side. That would produce a zero net force (though possibly a very slight torque).
But this is a problem because then we have a situation where one object is changing its momentum (the electron is going to curve in some direction due to the field), but no corresponding change in momentum of something else. Which isn't consistent with points we've spent a lot of time banging on in the intro courses.
I think the answer here is something to do with the momentum of the electromagnetic field itself, but I'm not sure there's an easy way to explain it. It's been so long since I took the sort of class where you would calculate that sort of thing that I don't even know where to start, let along how I would explain it in terms that first-year students would follow.
This isn't entirely academic-- I have one student in my class with a gift for asking really difficult questions, who had me explaining the self-energy of the electron in QED the other day. I can probably wave hands convincingly enough to get through if it comes up, but it's a nice reminder that teaching intro E&M umpteen times doesn't actually mean you understand everything in it perfectly.

The approximation that the wire is infinitely long can also rescue you here, because it means the current carries an infinite amount of momentum. So the current can cede some of its momentum to the electron and "lose" some of its own, but \infty - (finite) is still \infty. In the finite-length-wire approximation the field will be nonuniform along the wire's length, presumably clarifying how to cure this pathology.
Out of the page ... ?
Really? I don't recall seeing the finite wire solution in closed form, but isn't it likely to be just a set proportion of the infinite case?
Out of the page is the right answer for the original question.
There's a closed-form expression for the B-field of a straight wire of length L, measured at a point along an axis through the middle of the wire, that we discuss in class. A more general expression would get messy, but is probably doable.
Any momentum if the EM field must be capable of interacting with matter -in this case the wire. By virtue of its acceleration the electron is radiating some EM energy, also the current in the wire (moving charge), see's some mag field from the current of the electron, so there must be a counter balancing force.
Of course an infinite wire is absurd. A finite wire doesn't make a circuit, although it could form a connection between two capacitors whose charge state is being changed by the curent.i So we then have effects due to both finite spatial dimensions and finite time span, either/both of which probably make things messy.
Yay!
I seriously had to consider whether I was supposed to use the left or the right hand. It's been more than a decade ...
I'm pretty certain the momentum lies in the EM fields, though I'd be at a loss to come up with a intuitive explanation for what's happening her. If you want a sci-fi link, say that EM momenta is how solar sails work (and I assume they're covering the Poynting vector at some point?). Beyond that, if you really feel like calculating some integrals, the relevant potentials are here:
http://en.wikipedia.org/wiki/Li%C3%A9nard%E2%80%93Wiechert_potential
And I don't think the finite-ness of the wire has much to do with it, because as long as the wire is long and (roughly) of equal length above and below, the force from the electron's B field will only cause torque (the B field has cylindrical symmetry).
These courses were a very long time ago for me too, but I don't see why the electron's magnetic field being symmetric precludes the reaction force on the wire from being directly into the page, as you would expect from a naive conservation-of-momentum argument.
Ok.
I'm looking at this from the perspective of high-school physics, with a smattering of first-year introductory physics.
I can't see how you'd get the electron experiencing a force and acceleration out of the page.
Be nice to a poor chemist :)
Feynmann poses a puzzle like this with a solenoid, rather than a straight wire, and conservation of angular rather than linear momentum, in Lecture II.17.4. At the end of Chapter 27, he reveals that the answer is momentum in the field.
These courses were a very long time ago for me too, but I don’t see why the electron’s magnetic field being symmetric precludes the reaction force on the wire from being directly into the page, as you would expect from a naive conservation-of-momentum argument.
The reaction force is generally transmitted by the same mechanism as the action force. That is, when you're calculating the gravitational force on a falling object due to the Earth, you would say that the reaction force is the reverse of that. But you can get the same answer by directly calculating the gravitational force of the falling object acting on the Earth.
Or, for a less trivial example, a charge interacting with an electric dipole (equal positive and negative charges separated by a small distance) can feel a force that goes "sideways," parallel to the dipole rather than along the line between charge and dipole. But you can see the origin of this by working out the forces from the individual charges making up the dipole, and also see that there's a reaction force by calculating the effect of the single charge on the components of the dipole.
So, you don't just magically get a "reaction" force in normal situations-- those forces are the other end of a two-way interaction. But when you start dealing with magnetism, things get muddied a bit.
I can’t see how you’d get the electron experiencing a force and acceleration out of the page.
Be nice to a poor chemist :)
Unfortunately, the answer is pretty much "Because." That is, the magnetic force on a moving charge goes at right angles to both the magnetic field and the velocity, and that's just the way it is. Since the magnetic field in the picture above points upward at the location of the electron, and the electron is moving right to left, the only directions available that are perpendicular to both up and left are in and out of the page; in this case, it happens to be out.
This has lots of neat consequences, and plays a role in NMR, so it is important for chemistry. But I don't know of a really good conceptual explanation of why it needs to be at right angles to everything. It's just the way magnetic fields happen to behave.
.
I don't think a finite length wire (or even an arbitrary circuit) is that bad to compute. Biot-Savart reduces it to an integral over the current along the wire. If an analytic result doesn't pop out, accurate numerical integration isn't hard to do.
I think where I'm having issues is using the right hand rule to indicate the direction of the force.
If I use my thumb to indicate the direction of the current, then my curled fingers give me the direction of the induced magnetic field around the wire. I get anti-clockwise, as viewed on the page, therefore the field is pointing upwards as the electron enters it. So far, so good.
If I then use my right hand and aligning my index finger with the induced magnetic field, the middle finger with the current/electron movement, the thumb should indicate the direction of the force.
Looking at it, I get out-of-the-page.
Man, that definitely put a new wrinkle into my brain.
It just shows how much vector work I need to do :)
The original question was "what happens to the wire".
The answer is, the average (over a cycle) force will repel it from electron. This has nothing to do with momentum conservation:
If this electron were moving in the opposite direction, it would be repelled anyway.
Alex, I believe we'd be assuming the wire is carrying a DC current, so there are no cycles to consider. At the given time, as Chad notes, there's going to be a torque on the wire but not not a net bulk force.
The infinite wire would probably be more easy to compute, as imposing a length limit just means that you have some small term at large |z| to deal with instead of having something integrating to zero.
Whoops, I think I misread how you describe cycle. Since the B-field decreases inversely with distance from the wire, I don't believe there will be any truly closed orbits of the electron--you'd either have some spiral-like structures or probably something like a hyperbolic orbit of the electron (if it's moving over a distance where the B-field changes considerably).
In the problem as posed, all orbits would be finite, the integral of 1/r is the log function which is unbounded as you approach infinity. For small velocities the orbit would be nearly circular, but with a drift velocity imposed, because the radius of curvature is higher closer to the wire. Because the accelerating electron emits E&M waves, the radius of these smaller orbits would decay with time.
@tcmJOE: If the magnetic field is sufficiently strong that the Larmor radius of the electron is much smaller than its distance from the wire, then we can average the motion over a gyration period (which is what I think Alex means by "cycle"). In this limit the electron can be viewed as gyrating about a "guiding center" which moves into the page: because the magnetic field gets weaker as you move away from the wire, the electron travels farther on the portion of the orbit where it is moving into the page than the portion where it is moving out of the page. (See "gradient drift" in any plasma physics textbook.) This produces a net current out of the page due to the motion of an electron, which as Alex notes produces a force pushing wire and electron apart.
I don't know what the result is if you can't make the guiding center approximation, but if the Larmor radius is larger than the initial distance between electron and wire, the electron will crash into the wire (assuming gravity is negligible, as it usually is in these problems).
Ah, y'all are correct. Grad-B drift is perpendicular to both B and gradB, so the electron should ultimately drift downward (leading to an upward current). Thanks for the correction!
I think the problem is that, from the perspective of the wire, the moving electron is not in a magnetostatic state.
In other words, The electric field created by the electron at the location(s) of the current is changing, so you have to introduce that annoying dE/dt term to Ampere's law to figure out the total force
Chad, I had an undergrad ask me an equivalent question this past fall when I was teaching mechanics. Purcell talks about this issue of momentum being transferred to and from the fields. Unfortunately, as you point out, it is very hard to come up with a clear "equal and opposite" force when you are dealing with magnetic fields.
Out of the page is the right answer for the original question.
I thought the right hand rule would say that the thumb is sticking up out of the page and the magnetic field would be oriented as the fingers are so that the electron would move to the top of the page, not out of it. It's been 20 years since I've been in a physics class, sorry.
@Juice: That is indeed the correct orientation for the magnetic field, but the force it produces is orthogonal to both the particle velocity and the magnetic field (F = qv X B, where I'm using X to mean the cross product). For an electron (negative q), this force is out of the page.
Ah! Yes, ok. Thanks, Eric.
Basically, apply right hand rule twice.
Easy answer: The net force on the current in the wire is into the page. We know this must be true from good-old "equal and opposite".
Explanation: This force must arise from fields of the electron acting, of course. Calculating this is tricky (otherwise you wouldn't have asked). Perhaps the simplest way to see it is to transform into the inertial frame where the charge carriers in the wire are stationary. In this frame, the electron is moving "diagonally" towards the wire (rather than purely radially).
This frame is nice because now I don't have to worry about magnetic fields, since the current carriers are stationary: the only thing that can give a force is the electric field of the diagonally-moving electron. You MIGHT think that the net electric force is zero, but that's because you're ignoring relativity (which you must for any magnetic effect). Including relativity, the field intensity is NOT uniform as a function of the angle with respect to the direction of motion (see the beautiful pictures in Ch 5 of Griffiths), and you get a net force into the page. I wouldn't want to do the integral to show that it's the same magnitude as the force on the electron, but it's pretty easy to convince yourself that there will be a net axial field giving a force into the page.
(Note there will also be a radial component, but if we have a conducting wire, surface charges will form to cancel this out.)
Edits: "fields of the electron acting" should be "the fields of the electron".
"which you must for any magnetic effect": should be "and you need to include relativity whenever calculating magnetic forces"
Sorry about the typos, and it's hell trying to explain this without drawing pictures and waving my hands, but I think this is correct. At least I hope so: I just got done teaching a semester of undergrad E&M!
If instead of the wire you had a stream of electrons, (opposite the original current, what happens to the entire setup? Does the original electron even move the same way?
If you restrict the stream of electrons to a straight line, will it still be a momentum sink in the right direction?
The approach described by Anonymous Coward @27 is what is done (for simpler situations) in Berkeley Physics vol. 2.