The Shadow Knows

My last post was political, and to be quite honest I sort of hate it when my favorite non-political writers decide to break out the soapbox and flog their views in public. Since I have just done so, let's at least partially make up for it by another post talking about something near and dear to my heart - the propagation of light. In this case, let's talk about shadows. Here's a cool one from Wikipedia:

The basic idea of a shadow is simple. You have a light source, rays of light come out of it, some of the rays hit an obstacle, and the shadow is the region where the light has been blocked.

This intuitive idea of a shadow relies on the idea that light travels in straight lines, as though it were made of a stream of little particles. This was the idea of light that Newton championed, and in many respects it works pretty well. But thanks to the efforts of people like Huygens and Maxwell, we now know that light is in fact a wave phenomena. Waves can propagate around obstructions. Again, from Wikipedia:

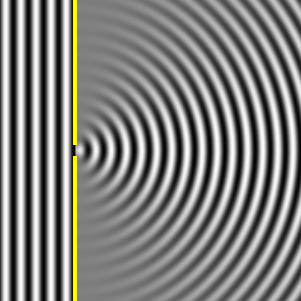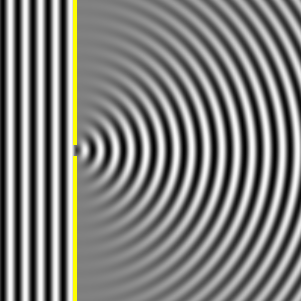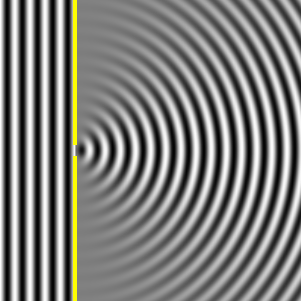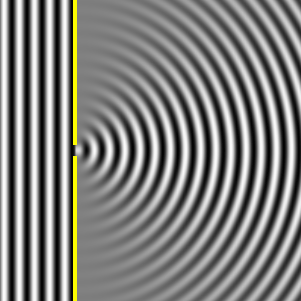
The light is coming in from the left, and the "shadow" - the region immediately behind the walls - does in fact not have much light in it. But farther out, the light diffracting from the opening starts to fill space. Why doesn't this happen with the shadows we see?

Well, it does. The reason we don't usually notice is because of the large scale of the obstructions relative to the small wavelength of the light.. Notice in the previous paragraph how I said "the region immediately behind the walls". This is slightly vague, but it's the region where light can be treated in the "ray optics" approximation where shadows work as we traditionally expect them to. But we don't want to be vague - so what counts as "immediately behind the walls"? Microns? Kilometers? It turns out that there's a figure of merit that tells you exactly what counts, and it depends on the size of the object and the wavelength of the light:

$latex F = \frac{a^{2}}{L \lambda} &s=1$

F is called the Fresnel number, and it tells you if you're in the region where the ray optics approximation works or not. If F is much larger than 1, ray optics works well. If it's much less than 1, you need to use the more accurate wave optics description. Here a is the size of the object making the shadow, L is the distance from the object to the screen, and λ is the wavelength of the light. Take the example of a person of size a = 2 meters standing L = 5 meters from a wall. Under these conditions, F is more than one million and shadows look like they're supposed to. Are there any easily visible cases where F is small?

Sure. One of the easiest demonstrations is a holding a hair in front of a laser pointer. A hair is perhaps a hundred microns in diameter, so if you hold a hair in front of the laser and shine it at a wall two meters away, the Fresnel number is just about 0.01. The shadow approximation doesn't work at all in this regime. So you won't see a simple line shadow - you'll see something that looks a lot like a single-slit diffraction pattern. Try this with the laser at different distances from the wall and see the pattern change as the Fresnel number changes.

More on this next week, where we'll look in a little more depth at how to calculate what shadows/diffraction patterns look like in the intermediate regime around F = 1.

[Update: I have revised some wording in the paragraph above the equation to make it more clear.]