Sunday Chess Problem

Our diagram position today was composed by Joseph Babson in 1927. The stipulation is selfmate in three:

Recall that in a selfmate, white plays first and forces black to give checkmate. That's right! White is trying to get checkmated, while black is doing everything in his power to avoid giving checkmate (or at least to postpone it beyond the stipulated number of moves). It's a complete inversion of normal chess logic.

Let's get right down to business. The key move is for white to play 1. a8Q!, leading to this position:

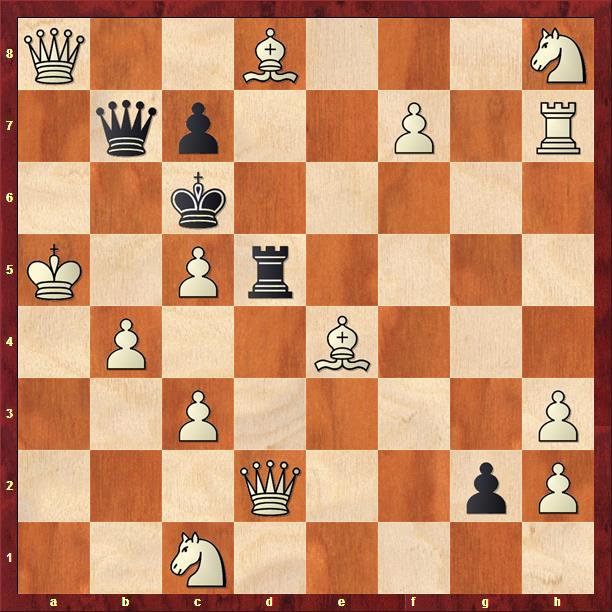
Folks, as key moves go, that's pretty appalling. Promoting to a queen is an incredibly strong move to begin with, and in this position it has the effect of completely tying black up into knots. What kind of fireworks could possibly be sufficiently impressive to compensate for such a ridiculous key move?

Wait and see!

The basic mechanism is now clear. If black plays 1. ... Kd7, then white achieves his goal with 2. Bf5+ Kc6 3. Qxg2 Qxa8 mate. Black's only other move involves promoting his pawn on g2. So, white's idea is this: After black promotes his pawn on the first move, white must be prepared to capture it. For reasons that will shortly become clear, he will achieve this by promoting his pawn on f7. However, black can promote to any of four different pieces. White's decision of which piece to promote to will be governed by black's choice of promotion.

For example, if black plays 1. ... g1Q, then it turns out that white must reply with 1. ... f8Q:

Let's see why. If black now plays a random move, like 2. ... Qg3, then white simply takes it with 3. hxg3, after which black is forced to give mate with 3. ... Qxa8 mate. But what if black plays 2. ... Qxc5+? In this case white has 3. b5+! and black is now forced to reply with 3. ... Qxb5 mate:

And now you see why white could not have promoted to a rook or a knight. Such a promotion would have left d6 unguarded in this line. After white's 3. b5+, black escapes with 3. ... Kd6.

If white had promoted to a bishop, then d6 would remain guarded. However, black now escapes with 2. ... Qg8:

White has no way to take the queen.

Let's move on. If black plays 1. ... g1R then white must reply 2. f8R.

Now, after almost any move by the black rook white will simply take it, after which black will once more be forced to give mate with 3. ... Qxa8 mate. The reason for white's rook promotion does not become clear until black tries 2. ... Rf1. Had white promoted to a queen instead of a rook, he would now be forced to play 3. Qxf1, leading to this position:

Do you see the point? Black is once again forced to play 3. ... Qxa8+, but this is not mate since white can interpose his queen on a6. With a white rook sitting on f1, this option is not possible.

Incidentally, in the line with the queen promotions, had black tried to take advantage of this option with 2. ... Qf1, then white does not reply by taking the queen. Instead he once more plays 3. b5+, which forces the reply 3. ... Qxb5 mate.

Perhaps you see the incredible pattern that is unfolding. If black plays 1. ... g1B then white must reply with 2. f8B:

This time the key line occurs after 2. ... Bxc5 3. Bxc5:

And now black is once more forced to give mate with 3. ... Qxa8. Had white promoted to a rook or a knight instead, then after 2. ... Bxc5 he would be forced to take with 3. bxc5. Now 3. ... Qxa8 is no longer mate, since white's king has acquired a flight square on b4.

Finally, after 1. ... g1N white must play 2. f8N:

The point of this promotion becomes clear after 2. ... Nxh3 3. Rxh3,:

Had white promoted to anything other than a knight, then the black king could now escape to d7.

That's pretty sweet! Previously in this series we mentioned that a problem in which all four of the possible pawn promotions take place is said to show Allumwandlung, usually abbreviated AUW. In this case we have double matched AUW. In each line, white must promote to the same piece as black. A problem exhibiting this idea is said to exhibit the Babson task.

Underpromotions, by which I mean a pawn promotion to anything other than a queen, are a perennial favorite for problem composers. In a regular game of chess, there are only a few reasons for considering an underpromotion. You might promote to a knight instead of a queen, since a knight can do things a queen cannot do. The only reason for promoting to a rook or bishop, however, is to avoid stalemating black.

In selfmates, however, underpromotions are somewhat easier to force. White must avoid being too strong, after all. That idea is well illustrated in the rook promotion line above, when promoting to a queen would have allowed white to avoid checkmate. That motivation does not exist in regular chess!

For this reason, perhaps it is not surprising that the Babson task was first achieved in selfmate form. The question is this: Is it possible to achieve the Babson task in a regular, direct mate problem? For many decades, problem composers tried and failed to accomplish it. It was commonly thought to be impossible.

But if that were really the end of the story, I wouldn't have bothered telling you all this! Tune in next week to find out how the task was finally accomplished...