One of the simplest tricks you can use physics for is to figure out how high up you are. Either using a stopwatch or just by counting seconds, drop a dense object (e.g., not paper, a tissue, etc.) and figure out how long (in seconds) it takes from when you release the object to when it hits the ground. Take that number and square it. Multiply it by 16 to get your approximate height in feet, or multiply it by 5 to get your approximate height in meters.
What's remarkable is that all objects fall at the same rate. All of them. It doesn't matter what material they're made out of, how large or small they are, or even whether it's moving horizontally or not!
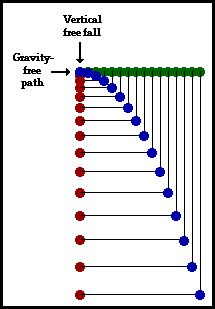Image credit: U.C. Berkeley, via http://cfpa.berkeley.edu/Education/ISTATPage/MiddleSchool/accelerations….
There's only one thing that can screw this up: air resistance. If we were doing this experiment on a planet with no air, like Mercury or the Moon, this method would always work. But Earth has an atmosphere, and if the object you drop is either going too fast or its density is too low, you start running into every falling object's nightmare, terminal velocity.
Terminal velocity is the speed where the drag force, or the force pushing you up from moving through the air, balances the force of gravity. Fall slower, and gravity will accelerate you up to terminal velocity. Fall faster, and air friction will slow you down to terminal velocity. A human without a parachute in the pencil position falls at around 160 miles per hour (250 kph), while one with a parachute has a terminal velocity of about 15 miles per hour (24 kph).
Now, there is a famous story that goes along with the discovery of the relationship between the distance something falls and the time it takes it to fall. Galileo allegedly went up to the top of the Leaning Tower of Pisa -- the tallest building he had available to him -- and dropped two different balls off of it; one 10 pound cannonball and one 1 pound ball of the same material.
According to the legend, they hit the ground at exactly the same time. But would they? Let's figure it out. A 1 pound ball has about 22% of the surface area as a 10 pound ball of identical material, and about 22% of the drag force of the 10 pound ball. But it has only 10% of the force of gravity! This means that as the ball falls farther and farther, the smaller mass ball starts to fall behind, since its drag force is a larger fraction of the gravitational force pulling it down. If we went up to an infinite height, the terminal velocity of the large ball would be 215 mph (340 kph), while the small ball would only reach 145 mph (235 kph).
The Leaning Tower of Pisa is tall -- about 55 meters (180 ft) high -- but things are moving much slower than terminal velocity. With a little math (it takes some to include air resistance), I can figure out that both balls would have hit the ground after just over 3 seconds of free fall. But not only is there a difference in time, the smaller ball hits the ground more than a quarter of a second later than the large ball! At this height, that means the small ball is over 20 feet behind the large one at impact! It'd be like watching Usain Bolt run a 100 meter race against normal men:
So what do we learn from this? In real life, different balls fall at different rates! It's the fault of the air, sure, but the tiny little bit of atmosphere we have is enough to totally throw even the simplest physics experiment off! And historically, we can learn that Galileo never actually did this experiment, because if he had, he would have found that his predictions were wrong! In real life, different balls don't fall at the same rate.
I'll repeat that: Galileo never actually did his most famous experiment! Go to such great heights and try it for yourself!
- Log in to post comments
More like this
The Leaning Tower story comes from (IIRC) an assistant of Galileo named Vincenzo Viviani, who wrote about it in a biography written well after Galileo's death. No one else is known to have recorded the incident in a journal, which makes the story that much less likely to have occurred. Cool story, though. Even cooler is David Scott's verification of the principle on the Moon in 1971.
Boo! I like the Galileo story!
This reminds me of a mechanics lecture I went to where the lecturer recreated the monkey-and-the-hunter experiment. He suspended a teddy bear from the top of the lecture theatre and aimed an air cannon at it (with a laser sight no less!) and simultaneously released the teddy and fired the cannon.
Think he got a standing ovation for that one...
Doug, poor show for not including a link for something that sounds as interesting as "the monkey-and-the-hunter experimnent" :) Though it may be that everyone else has already seen it.
http://www.youtube.com/watch?v=cxvsHNRXLjw&feature=related
At least the story about Galileo and Usain Bolt hitting the ground at the same time after falling off the leaning tower is true. Right? Maybe I heard it wrong.
I did my A-level physics coursework on the bouncing thing.
That wasn't supposed to be the purpose, we were supposed to measuring how the properties of a plastic were affected by temperature. As part of that, it was required to measure how much kinetic energy was lost when a ball heated to the required temperature was bounced from a known height to a perpendicular surface. A simple matter of measuring it's velocity, but in turn an engineering problem: No high-speed cameras, and the ball wouldn't bounce quite straight enough to always hit the very narrow sense region of a light gate.
The solution of all the other students was to bounce it vertically, and try to see on a scale how high it went. Very inaccurate: They could get it's height to within 5cm at the most.
I attached a microphone to the surface, and recorded the sound on the computer. Drop. Bounce. Pause. Bounce. By measuring the exact time between the bounces (down to a few miliseconds) I could calculate just how heigh the ball bounced, and from that calculating either it's kinetic energy or velocity was easy.
I still got only an average grade, though, due to the shortness of my report... it consisted of three pages, one of which was just the derivation of the equasions used. Expectation, diagram of apparatus, raw data, analysis, conclusion. That was it. Most of the students produced thick wads of paper that detailed every tiny detail of the experiment and the background science we were investigating (Then a page explaining why their data looked like random noise due to the extreme inaccuracy of eyeballing a moving object against a scale). At A-level, pages mean marks - the more you write, the more potential places there are for ticks to be placed.
Both ball would have hit the ground at the same time if tone was an iron cannonball and the other a wooden ball of the same volume, hence the same drag.
Typos fixed: Both balls would have hit the ground at the same time if one was an iron cannonball and the other a wooden ball of the same volume, hence the same drag.
Arthur,
Although the drag force would be the same, the force of gravity would be different, so no, they would hit at different times.
I remember asking my physics teacher how they managed a vacuum outside the Tower Of Pisa when Galileo dropped the ball. I remember doing extra credit homework for the next day, as well.
The would only hold true if weight and volume were tied to size. How would the math work for that?
as weight increases, volume also? Someone who can do math show me how to write that out?
Thanks for pointing out the urban legend - and that's a fantastic photo of the bell tower - it's even got part of the church in it! (Thus dispelling the strange notion many tourists have that there's this marble tower sitting out in the middle of nowhere ...)
This statement has thrown me off though since I can interpret it several ways (all of which are incorrect):
"But it has only 10% of the force of gravity!"
The drag is roughly proportional to the speed of the object and its exposed surface (ah, if everything were as simple as a sphere). The object will decelerate according to its momentum and drag, and accelerate according to gravity.
What I don't get is why the smaller object would only have "10% of the force of gravity" when the gravity in either case is obviously vastly dominated by the earth's mass.
When I was a little kiddie, my books told me that Galileo rolled things down a ramp; the dropping cannonballs from the tower of Pisa was never mentioned. I wish I could remember what book that was; I think I was too young to understand the explanation of the ramp experiments at that time.
matt,
For objects of the same shape and uniform composition, their weight is proportional to their volume, which is proportional to the cube of their length. The drag force on them is (roughly) proportional to their surface area, which is proportional to the square of their length.
x^3 gets big faster than x^2, so for bigger and bigger objects, weight dominates drag more and more, and the object falls faster. In the limit, it falls as fast as it would in a vacuum.
Slight correction--it'll only decelerate due to drag, not due to momentum. Momentum is not a force, but a measure of what forces have been doing to the object over time. (So you could also say that gravity will increase the object's downward momentum, and drag will decrease it.)
What I don't get is why the smaller object would only have "10% of the force of gravity" when the gravity in either case is obviously vastly dominated by the earth's mass.
The gravitational force between two objects is proportional to the product of their masses, so the gravitational force on an object near Earth is equal to
constant * mass_earth * mass_object. For the smaller object, mass_object is 10% of what it is for the larger object, so the whole product is also 10% as much.
Years ago I read an article about a parachute jump master whose mascot cat fell out of the plane from 3000 feet and lived.
So he got a group of alley cats and tossed them all out of the plane from 3000 feet and they all lived. One even came back for more.
So a physicist friend and I made some assumptions about furry cats with legs and tail splayed out in terror and how much drag they might create and came up with a feline terminal velocity.
We decided the story was plausible.
After all, have you ever heard of a cat getting hurt in a fall?
Ethan: "Although the drag force would be the same, the force of gravity would be different, so no, they would hit at different times."
Uh? The acceleration due to gravity would be the same, hence the whole point of Galileo's apparent experiment. They'd arrive simultaneously.
They would make different size holes in the ground, however, since the larger would have more momentum, due to the larger force on it.
Davem: the acceleration on the object falling is a result of the force exerted by gravity and the amount of resistance the object experiences while falling. The 9.81m/s^2 is the acceleration at sea level in a vacuum, not the falling acceleration of all objects near the earth. To illustrate this, consider a dirt road. The fine dust and the large rocks are all composed of the same material, yet the rocks fall back to the earth very quickly after they've been kicked up, while the dust lingers for some time before settling. The same thing happens with the cannon balls, except that the difference in drag is far less pronounced than with the dust and gravel. Another way to consider this is by thinking about the relative speed that large and small stones sink through water with, but there the difference is more pronounced because the fluid (water as opposed to air) is denser and causes more resistance to the falling objects. On planets where the atmosphere is much denser yet, the resistance goes up accordingly, so that a falling object in Jupiter would fall far slower than the planet's gravitation would allow.
Galileo apparently figured out the isochrony of the pendulum by seeing a swinging chandelier (maybe an incense holder) in the Cathedral - the church you can see in the pictures.
Is that also false?
I think the point of the experiment is that the difference in falling times is very small as compared to the difference in weight of the balls. Aristoteles had compared things like stones and feathers.
Estraven: I'm not sure about the pendulum story, though I can't think of any similar reason why it would have to be false. As for Aristotle, I can't help but do a facepalm for humanity with him, and not just for stones and feathers, but the whole essentialism vein of thought leads to so many clearly impossible logical outcomes, especially when it gets around to giving support to sympathetic magic. But even now, long after atomism is proven and thus homeopathic and contagious magics are impossible fundamentally, so many people continue to believe in them.
Sorry, that was pretty badly off topic.
You have an awesome blog going here dude. I love it.
davem,
But gravity is not the only source of acceleration. There's also drag, acting against gravity, and the acceleration contribution from drag is greater for the lighter object. (Acceleration is force/mass, so the same drag force on a lighter mass means more acceleration.)
estraven,
Yep. The swings of a pendulum are not isochronous; they take longer for larger swing angles. This becomes easily noticeable for swings past 15 degrees or so from vertical. As the maximum pendulum angle from vertical approaches 180 degrees, the time it takes to complete a swing increases to infinity. At 180 degrees, the pendulum's balanced straight up and it never falls down at all!
Raimund,
I tend to blame Plato more for essentialism; Aristotle just continued that line of thought. Anyway, I think Aristotle more than redeemed himself by, y'know, single-handedly founding multiple sciences and popularizing the idea of empirical research and all. It's not his fault a bunch of folks 1500 years later decided first that he was wrong about everything (the medieval Church initially considered support of "Aristotelianism" heretical!) and later that he was right about everything.
This is of really great interest to me and my readings in the history of science have all suggested that the Leaning Tower story is indeed apocryphal.
What I have not come across, however, is the definitive piece of historical scholarship which documents this. Does anybody know what it is?
OK, second attempt at a post (I hate computers today).
@Anton Mates: You're right about the momentum; I should have written 'mass' instead; stoopid me.
I also see now where I got confused; when Ethan wrote "force of gravity" he really meant it: F = m*g
I have a very bad habit developed from people misusing that phrase and saying "force of gravity" when they mean "gravitational acceleration". In person if someone says "force of gravity" I usually start moaning and tearing out my hair because I know they don't mean it. So, despite a few confused readers, Ethan's article is correct.
Sorry, Ethan. I think you are wrong.
Density of wrought iron = 7850 kg/m3
It can be calculated that the cross-sectional areas of the 10 and 1 lb balls are, respectively, 13.01 and 2.80 square inches.
A ball has a drag coefficient of 0.25 to 0.28. Using the 0.25 figure and common equations (ok -- I cheated and used the NASA calculator at http://www.grc.nasa.gov/WWW/K-12/airplane/flteqs.html) for the 10 lb ball, I get 3.365 seconds to fall the 183.27 feet, with a velocity of 109.5058 ft/sec when it hits the ground (far slower than the terminal velocity of 610.4 ft/sec)
For the 1 lb ball, I get 3.354 seconds to fall the same distance, with a velocity of 110.52 ft/sec when it hits the ground (far slower than the terminal velocity of 416 ft/sec)
I have a difference of 0.011 seconds between the two.
This ends with the smaller ball being 1.2 feet behind the larger one.
0.011 seconds, I would guess, is at the threshold of human perception, and would be defined as "simultaneous" by any observer.
No fudging here, I think.
@Chris C. Mooney: There is no direct proof, but basically there is no documentary evidence of Galileo performing such an experiment, not even any entries to that effect in Galileo's notebooks.
Galileo was very intelligent and very practical; when confounded by his inability to time falling objects to his satifaction, he worked out that he could get the information he wanted by rolling things down a ramp. Rotational inertia became an issue then and he also recognized that. Galileo also used the position at which an object started on his ramp to control its speed at the bottom of the ramp. This allowed him to gather data on the position of his lead shot or marbles or whatever he used, and he demonstrated that the "obvious" intuitive claim of Aristotle about projectile motion was absolutely wrong. An object did not travel in a straight line until above a point then drop; a (small) projectile moves with the same horizontal velocity and at the same time falls toward the earth just as a dropped object would. (Personally I can't imagine how anyone who had seen an arrow in flight could believe Aristotle.) Would someone of Galileo's intellect, someone who could invent such techniques for measuring acceleration and who could also see the problems with his techniques, someone who's inevitably seen feathers and leaves falling slowly to the ground and stones falling rapidly, really bother to drop two different sized canonballs from a bell tower? It is a believable situation, but there is no evidence for it.
The air resistance/drag force is NOT the only thing making objects fall at different speed.
The density matters - because of the Buoyancy force (aerostatic lift). A stationary balloon experiences no air resistance (only moving objects experience a drag force from the air), but still stays afloat.
Given two balls with the exact same shape, but different density, the heaviest one will reach the ground first.
Objects only fall at the same rate in vacuum.
@Chris C. Mooney: You can try sections of Lane Cooper's "Aristotle, Galileo, and the Tower of Pisa" for a start; Cooper draws on other people's translations and comments on the matter. Someone did conduct such an experiment and wrote to Galileo to ask for help in interpreting the results (statisticians love it when someone runs their own experiment then comes looking for them to interpret it) but it seems clear that Galileo didn't recommend such an experiment much less carry one out as claimed in the myth.
I think the problem here is that we are talking about two different experiments. One uses two balls of the same material (iron), but at 10 lbs and 1 lbs. Thus they would have the same density, but different volumes.
The other is using two balls of the same size, but different material (iron v. wood). Thus they would have the same volume, but different densities.
The two different sized balls of the same material would land at almost (but not quite) the same time. With the same sized balls, the heavier one would land much sooner.
If Galileo had done his experiment with two different sized iron balls, it would have appeared to observers that they landed simultaneously.
Right?
@Chris,
Raj's comment (the first one) about the origin of the story is, as far as I know, correct. It seems that the only accounts are secondhand and later than Galileo, but I can't find the research myself.
@Warren,
I get the drag coefficient to be 0.31, but the important part that you're missing in your equation is that velocity as a function of time is dependent on both the cross-sectional area and the mass of the object. The smaller mass object falls more slowly, and takes more time to hit the ground than the large mass one.
In any case, you would definitely see the heavier object strike the ground first from a height of 55 meters.
I heard (and watched a recreation of) the "rolling down a ramp" variation in my college physics classes. If memory serves, they were two cylinders of uniform density. We also did the variation where the two cylinders had the same mass but differing radial density: one was solid wood, the other was a ring of metal. (That one demonstrates rotational inertia; the wooden cylinder reaches the bottom of the ramp faster, since the metal ring has to overcome more inertia.)
To the casual observer, the two cylinders of differing weights reached the bottom of the ramp simultaneously. I'm sure that there was a difference in drag, but the effect of that difference was negligible compared to, say, the timing issues with releasing both cylinders by hand.
Matt Springer at Built On Facts also has a writeup of the Galileo experiment, and defends it, despite its shortcomings.
Re: pendulum experiments
Yep. The swings of a pendulum are not isochronous; they take longer for larger swing angles. This becomes easily noticeable for swings past 15 degrees or so from vertical.
In the pendulum the restoring force is proportional to sin(θ) where θ is the angle from vertical. For sufficiently small angles we can approximate sin(θ) as θ (if we are measuring angles in radians). More generally, sin(θ) can be written as the sum from n = 0 to infinity of (-1)^n/(2n+1)! * θ^(2n+1), although practically speaking you would not evaluate this series for θ larger than 1.
Y'know, I've got a really silly suggestion for this dispute: why doesn't someone try it? I'm sure someone can find 11 pounds of iron and a 55-meter height to drop them from. Film the release and the impact (for comparison) and see how much air resistance affects the outcome.
But 15 degrees is a pretty large displacement for a heavy chandelier.
It doesn't seem unbelievable that GG woulda noticed small swings during a boring sermon.
Of course, being believable is what makes an urban legend.
I do believe some Dutch physicist actually did try dropping balls from a churchtower around that same time. I forget the name, though.
Right: as the angle of the pendulum's swing increases, the time increases, assuming a rigid arm.
I was told, however, that a "pendulum" with a flexible 'arm'---rope---and catenary-shaped guides would be isochronous. Is this correct?
Sili:
Simon Stevin.
@Jodie, actually a common injury for cats falling from height is a smashed jaw. I.e. the cat lands on its feet OK but the head continues downward with the lower jaw impacting the floor.
Fascinating post, with a big "But" (I'll get to that in a sec). I always assumed the Pisa thing was apocryphal, because it just kinda sounds ludicrous -- but I always assumed the experiment would have more or less worked, because the air resistance would have been negligible.
And now... But I really, really, really want to see a video of somebody actually doing this experiment. I guess it's because I never had to take enough physics to really get an intuitive grasp of air resistance (me=computer engineer, so we went as far as a 10-week combined crash course on statics&dynamics, as well as a 10-week modern physics course to just scratch the surface of relativity, etc.... so I know a fair amount about physics, but when you get to the nitty-gritty of anything beyond basic Newtonian stuff, I'm pretty lost). It seems intuitively wrong to me that the air resistance would have that much of an effect at that height -- which is not to say I doubt your conclusions, but only that I would find it edifying to see the experiment actually done! :) :)
Ethan, DataJack, you got it wrong.
If we drop 2 balls of the same volume, but different mass, they will hit the ground at the same time.
They both will accelerate at 9.8 meter per second squared. Notice that this equation has no mass variable.
9.8 m/s2, minus the drag, which will be the same for both balls.
And I endorse Warren Smith comment, the difference of drag between a 10-pounds cannonball and a 1-pound one would have been small enough to lead Galileo to the wrong conclusion, or, in this case, the right conclusion based on an erroneous observation.
It's worth noticing that a very slight difference in release times of the two objects will result in a large difference in the time of their landings.
How precisely can you (or Galileo) get the balls to be released simultaneously?
_Arthur: You are right - no equations for me before coffee. The ball's mass in "F=ma" and the ball's mass (m2) in F=(G*m1*m2)/r*r) cancel out. That just leaves you with the Earth's mass as the accelerator. That leaves us with a=G*m1/r*r. If we then plug in the mass of the Earth for m1, and the radius of the Earth for r, we get your 9.8m/s/s. In my defense, I have had more years since HS physics than I had before. *sigh*
I'm going to need a follow-up post to set this straight, aren't I?
Sili,
Oh yes, that part is entirely believable. It's just pretty unlikely that he followed it up with an experiment of his own, or he would quickly have noticed the discrepancy for large angles. (Unless he chalked it down to air resistance or something.)
_Arthur,
But drag is a force, not an acceleration. To get its contribution to the acceleration, you have to divide by the mass, which is not the same for both balls.
So the downward acceleration is 9.8 m/s2 minus drag/mass. Heavier ball has larger mass, hence that subtracted term is smaller, hence it accelerates faster.
Mikael,
You're perfectly right. I'm not sure whether buoyancy makes a human-eye-detectable difference in the behavior of these balls, but it certainly does make some difference.
Okay, help me please, Ethan. we say that objects all at the same pace all things being equal other than mass. They are attracted by Gravity, presumably.
F = GMm/R²
If R is the same, doesn't a bigger m (or M) get a bigger F, and so should fall faster. Would a black hole fall faster than a moon, if you get my drift?
Thanks!
Rich: Yes, the larger object gets a larger force applied, but not a larger acceleration. f=ma, so for, say, double the mass, gravity will exert double the force. Twice the energy is put into accelerating the heavier falling object. But the acceleration, and hence the speed, is identical. At the end of the acceleration period, the heavier object has twice the mass, hence has twice the momentum, and twice the energy.
My first comment was re Ethan's statement on the two balls of the same size: viz: "the force of gravity would be different, so no, they would hit at different times."
...which is plain wrong. The extra force on the larger object is all used up because it is accelerating a proportionally larger mass.
Rich,
How fast you fall is a matter of acceleration, rather than force. Acceleration is force over mass. A bigger m does give you a bigger F in that equation, but their ratio doesn't change, so you get the same acceleration.
From Galileo's _Two New Sciences_:
SIMPLICO: Your discussion is really admirable; yet I do not find it easy to believe that a bird-shot falls as swiftly as a cannon ball.
SALVIATI: Why not say a grain of sand as rapidly as a grindstone? But, Simplicio, I trust you will not follow the example of many others who divert the discussion from its main intent and fasten upon some statement of mine which lacks a hairsbreadth of the truth and, under this hair, hide the fault of another which is as big as a ship's cable. Aristotle says that "an iron ball of one hundred pounds falling from a height of one hundred cubits reaches the ground before a one-pound ball has fallen a single cubit." I say that they arrive at the same time. You find, on making the experiment, that the larger outstrips the smaller by two finger-breadths, that is, when the larger has reached the ground, the other is short of it by two finger-breadths; now you would not hide behind these two fingers the ninety-nine cubits of Aristotle, nor would you mention my small error and at the same time pass over in silence his very large one. Aristotle declares that bodies of different weights, in the same medium, travel (in so far as their motion depends upon gravity) with speeds which are proportional to their weights; this he illustrates by use of bodies in which it is possible to perceive the pure and unadulterated effect of gravity, eliminating other considerations, for example, figure as being of small importance, influences which are greatly dependent upon the medium which modifies the single effect of gravity alone. Thus we observe that gold, the densest of all substances, when beaten out into a very thin leaf, goes floating through the air; the same thing happens with stone when ground into a very fine powder. But if you wish to maintain the general proposition you will have to show that the same ratio of speeds is preserved in the case of all heavy bodies, and that a stone of twenty pounds moves ten times as rapidly as one of two; but I claim that this is false and that, if they fall from a height of fifty or a hundred cubits, they will reach the earth at the same moment.
It seems clear to me that Galileo did do the experiment, and he knew perfectly well that the two balls would not actually hit the ground at the same instant. He also understood that the issue was removing all non-gravitational influences, which he couldn't do -- so he emphasized how Aristotle's notion was just plain wrong because the dependence on mass had the wrong functional form.
He focused on the strong part of his argument, and didn't worry about the details until experimental technique could be improved to nail it down exactly. Sounds like a typical theorist to me (Hawking, quoting Eddington, "Don't worry if your theory doesn't agree with the observations, they are probably wrong.")
Next your going to tell us that Newton didn't "discover" the law of gravity after an apple fell on his head!
@Mark Betnel: You are imagining too much based on the evidence you have. The discussion makes it obvious that Galileo understood that objects more or less fall at the same rate but that objects with a greater drag (gold leaf, finely powdered stone) may settle very slowly, but even objects of different mass will more or less fall at the same rate with some observable discrepancy. The point of the argument is that Aristotle's mechanics of motion are simply wrong (99 cubits error vs 2 fingers' breadth). Nothing in the argument suggests that Galileo ever performed the tower trick, and we know full well that Galileo need not have performed such a stunt to come to the conclusions in that discussion.
Note that the claim here attributed to Aristotle can't actually be found in any of his surviving works (and it's rather unlikely, I think, that Galileo had access to more of Aristotle than we do.) See Aristotle, Galileo and the Tower of Pisa for more.
Thanks Aton and DaveM, I get it now (I think). There's a bit of 'cancelling out' going on!
@MadScientist
I should have been more specific when I used the phrase "the experiment" -- I don't think he actually dropped things from the Tower of Pisa, because I know, as you say, that he didn't need to. I do think that he probably did drop things from different heights and noted that Aristotle's position was toast based on what he saw, but was unsatisfied with the precision of the experiments, so developed the rolling-ball-on-inclined-plane technique.
I don't think Galileo would have been so foolish as to call Aristotle out for not having done the experiment if he hadn't personally done something equivalent himself. Assuming that he did, and that he saw what we see when we do it, I think it's not unreasonable to guess that he decided to disregard the discrepancy. But without further evidence, I suppose you could say that I am imagining this.
@Mark Betnel
Well done. Instead of speculating on what Galileo might have known you quote his writing. It's clear that he did know what the result of dropping two objects would be and had done some experiments about it. The clarity of his understanding is remarkable. It shines through the fog of translation and centuries of difference in learning and culture. The very heart of the scientific method right there in that short passage. Truely remarkable.
why does a body gets hurt when falls from a height(long)
why a person gets hurt when jumps from a height
I am a high-school freshman taking physics. My teacher asked that we name "who dropped weights from the tall tower (not pisa)? I get three tries, typed in Galileo (before reading this thread, it was wrong. Then input Philoponos but that was wrong, as well....any help would be greatly appreciated
Thanks
Well done. Instead of speculating on what Galileo might have known you quote his writing. It's clear that he did know what the result of dropping two objects would be and had done some experiments about it. The clarity of his understanding is remarkable. It shines through the fog of translation and centuries of difference in learning and culture. The very heart of the scientific method right there in that short passage. Truely remarkable. Christian Louboutin
Next your going to tell us that Newton didn't "discover" the law of gravity after an apple fell on his head! christian louboutin boutique
I do think that he probably did drop things from different heights and noted that Aristotle's position was toast based on what he saw, but was unsatisfied with the precision of the experiments, so developed the rolling-ball-on-inclined-plane technique.
yeah ,i think so,thanks for sharing,good job
Next your going to tell us that Newton didn't "discover" the law of gravity after an apple fell on his head! proofreading jobs
Thanks Aton and DaveM, I get it now (I think). There's a bit of 'cancelling out' going on! Louboutin
Well done. Instead of speculating on what Galileo might have known you quote his writing. It's clear that he did know what the result of dropping two objects would be and had done some experiments about it. The clarity of his understanding is remarkable funny stuff. It shines through the fog of translation and centuries of difference in learning and culture. The very heart of the scientific method right there in that short passage. Truely remarkable
I like to see the couple together in a picture as a sign of the test. I've never read about them to accept a relationship anyway.
One of the simplest tricks you can use physics for is to figure out how high up you are. Either using a stopwatch or just by counting seconds, drop a dense object (e.g., not paper, a tissue, etc.) and figure out how long (in seconds) it takes from when you release the object to when it hits the ground.Residential Skylights - Abba skylights supplies velux skylights for all residential skylights, industrial skylights, institutional skylights applications including custom glass skylights, energy saving/hurricane high impact strengthened skylights Take that number and square it. Multiply it by 16 to get your approximate height in feet, or multiply it by 5 to get your approximate height in meters.
One of the simplest tricks you can use physics for is to figure out how high up you are. Either using a stopwatch or just by counting seconds, drop a dense object (e.g., not paper, a tissue, etc.) and figure out how long (in seconds) it takes from when you release the object to when it hits the ground.Residential Skylights - Abba skylights supplies velux skylights for all residential skylights, industrial skylights, institutional skylights applications including custom glass skylights, energy saving/hurricane high impact strengthened skylights Take that number and square it. Multiply it by 16 to get your approximate height in feet, or multiply it by 5 to get your approximate height in meters.
I really enjoyed reading this post, big fan. Keep up the good work and
please tell me when can you publish more articles or where can I read
more on the subject? Thank you on behalf of the Administrative Resume team. we will really appreciate you for your upcoming blast
also.
One of the simplest tricks you can use physics for is to figure out how high up you are. Either using a stopwatch or just by counting seconds, drop a dense object (e.g., not paper, a tissue, etc.) and figure out how long (in seconds) it takes from when you release the object to when it hits the ground.Residential Skylights - Abba skylights supplies velux skylights for all residential skylights, industrial skylights, institutional skylights applications including custom glass skylights, energy saving/hurricane high impact strengthened skylights Take that number and square it. Multiply it by 16 to get your approximate height in feet, or multiply it by 5 to get your approximate height in meters.
One of the simplest tricks you can use physics for is to figure out how high up you are. Either using a stopwatch or just by counting seconds, drop a dense object (e.g., not paper, a tissue, etc.) and figure out how long (in seconds) it takes from when you release the object to when it hits the ground.Residential Skylights - Abba skylights supplies velux skylights for all residential skylights, industrial skylights, institutional skylights applications including custom glass skylights, energy saving/hurricane high impact strengthened skylights Take that number and square it. Multiply it by 16 to get your approximate height in feet, or multiply it by 5 to get your approximate height in meters.
One of the simplest tricks you can use physics for is to figure out how high up you are. Either using a stopwatch or just by counting seconds, drop a dense object (e.g., not paper, a tissue, etc.) and figure out how long (in seconds) it takes from when you release the object to when it hits the ground.Residential Skylights - Abba skylights supplies velux skylights for all residential skylights, industrial skylights, institutional skylights applications including custom glass skylights, energy saving/hurricane high impact strengthened skylights Take that number and square it. Multiply it by 16 to get your approximate height in feet, or multiply it by 5 to get your approximate height in meters.
"This ends with the smaller ball being 1.2 feet behind the larger one. 0.011 seconds, I would guess, is at the threshold of human perception, and would be defined as “simultaneous” by any observer." -- This best calculation is consistent with the 'two fingers-breadth' difference described by Salviati. The 'measurement' has significant systematic error, but suggests an actual observation of a slight difference -- supporting the claim that some experiment was done, if not exactly two cannon balls from the Tower of Pisa. The most important thing is the introduction of an error estimate -- that is what separates all of modern science from all of its predecessors in 'natural philosophy'.