When supernovae get too big!

Posted by Ethan on April 9, 2012
(31)
More »

“You cannot, in human experience, rush into the light. You have to go through the twilight into the broadening day before the noon comes and the full sun is upon the landscape.” -Woodrow Wilson

Without a doubt, one of the most spectacular light shows of the cosmos happens when stars burn out — reaching the end of their normal life cycle — and die in a great supernova explosion. We’ve spoken in the past about the main ways that these stars die. Either a very massive star — something more than ten times as massive as our Sun — reaches the end of its nuclear fuel, and its core collapses, blowing off its outer layers in a massive explosion.

Video credit: NASA / ESA, retrieved here.

This is how the Crab Nebula, from a type II supernova explosion nearly 1,000 years ago, was created. These supernovae leave behind a collapsed object, either a black hole or a neutron star, at the center of the now-destroyed progenitor star.

On the other hand, less massive stars don’t collapse like this; their core merely contracts as their outer layers are blown off much more gently. This produces a planetary nebula that fades over time, and a very long-lived white dwarf at its core.

Image credit: NASA, ESA and the Hubble Heritage Team STScI/AURA.

But these white dwarfs get a second chance at a supernova. When they either accrete enough additional mass or — as now seems to be the more likely scenario — merge with another compact object, they can also undergo runaway nuclear reactions. This typically destroys both stars, leaving neither a neutron star nor a black hole behind, but releasing a tremendous amount of energy in a type Ia supernova.

Video Credit: NASA / CXC / A. Hobart.

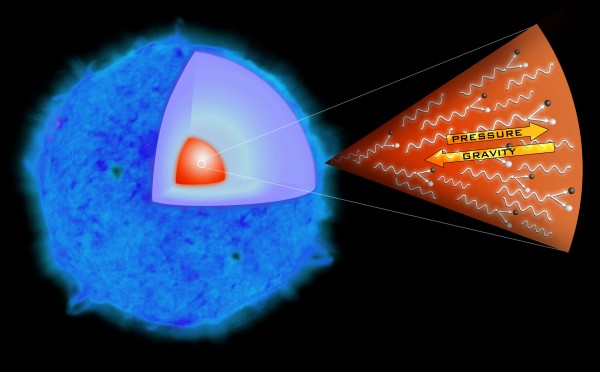
There is some variation among the core-collapse types of supernovae, but I’d like to remind you of what goes on inside of those stars. While nuclear fusion is occurring, the outward radiation pressure from the fusion in the core holds the rest of the star up against gravitational collapse.

Image credit: Sakurambo at wikimedia commons.

But when that nuclear fusion in the core runs out, the core collapses under its own gravity, emitting tremendous amounts of light via the conservation of energy. Why’s that? You know all about gravitational potential energy; it’s why that weight you drop onto your foot hurts so much! Well, when you collapse a large mass — something hundreds of thousands to many millions of times the mass of our entire planet — into a small volume, it gives off a tremendous amount of energy.

In theory, if we made a star massive enough, like over 100 times as massive as the Sun, the energy it gave off would be so great that the individual photons could split into pairs of electrons and positrons. Electrons you know, but positrons are the anti-matter counterparts of electrons, and they’re very special.

Image credit: NASA / CXC / M. Weiss.

Because a positron will run into an electron in short order, annihilating it, producing a gamma-ray. And if the rate of gamma-ray production is fast enough, you heat up the core. In other words, if you start producing these electron-positron pairs at a certain rate, but your core is collapsing, you’ll start producing them faster and faster… continuing to heat up the core! And you can’t do this indefinitely; it eventually causes the most spectacular supernova explosion of all: a pair instability supernova, where the entire, 100+ Solar Mass star is blown apart!

At least, that was the theory. Was, I say, because in 2007, that’s exactly what was observed!

Image credit: A. Gal-Yam et al. at the Weizmann Institute, Israel.

This supernova, known as Sn 2007bi, is exactly this type of pair-instability supernova that was only theorized. What’s extra remarkable about it is that, when we peer deep into the Universe to look at where it came from in depth, we literally see practically nothing!

Image credit: Sloan Digital Sky Survey.

I say practically nothing, of course, because there really is something there. Far away is a tiny, faint, and distant dwarf galaxy, just barely visible with the right image manipulation.

Dwarf galaxies, it turns out, form stars only on very rare occasions. But when they do, they form them in great bursts, with often the entire galaxy becoming a great star-forming region! When this happens — much like the great star-forming region at the center of our galaxy — we get large, 100+ solar-mass stars as part of the deal: the only candidate for forming these pair-instability supernovae!

As was just reported, this supernova, Sn 2007bi, is the first confirmed pair-instability supernova ever, and it needs this relatively pristine environment present only in young, dwarf galaxies (and not in our metal-rich Milky Way’s center) to do it!

Which means, of course, that if we want to see one close by, we need to know where to look. Ladies and gentlemen, may I present to you your neighbor, NGC 1569!

Image credit: ESA, NASA and P. Anders (Göttingen University Galaxy Evolution Group).

A dwarf galaxy so close to us it’s actually blueshifted towards us, this old, low-metal galaxy has been forming stars as recently as within the last 5 million years! (Look in the X-ray if you don’t believe me!)

So supernovae formed from massive stars will leave you a neutron star, or, if they’re bigger, a black hole, or, if they’re really bigger, absolutely nothing, except a much richer, heavy-element-filled Universe, perfect for creating things like you and me. And aren’t we fortunate for that!

(31)
More »

Comments

  1. #1 joe
    April 9, 2012

    Wow had the same lecture in class today

  2. #2 Justicar
    April 10, 2012

    You write herein that we should expect this pair instability supernova when we have a star circa 100 solar masses. I was under the impression that even 100 solar masses would not be sufficient (roughly a third below what is the minimum threshold) for this type of supernova, coupled along with the star’s composition. But assuming that you know more on this than I know (and I fully expect this is the case given this is *not* my field), and given that you recently wrote on Eta Carinae, the question immediately rushes to the fore of my mind: is Eta Carinae a likely candidate for this type of supernova? Or is its mass (and composition) over the range for this type, and thus a likelier candidate for a so-called hypernova?

    Looking on the ever accurate and scholarly wikipedia, I see that the mass range of 100 – 130 solar masses can have a collapse initiated by this pair instability, but that this pair instability is not ‘truly’ this type of supernova, for the pair instability within the star is not adequate to globally affect the star. For that, the 130 – 250(ish) solar mass range is necessary to consider the resulting destruction properly as this particular type of supernova. And, of course, the mind-boggling 250+ solar mass bracket, of which there seems to be at least candidate: R136a1, coming in with something on the order of 265 solar masses, and how that is expected to go the way of the dodo.

    Anyway, thanks for the article and food for thought (and something to spend some time researching). =^_^=

  3. #3 leticia Oyarzun Gomez
    April 10, 2012

    Wanderful, thank for to share.Leticia

  4. #4 Ron
    April 10, 2012

    Very informative read, thank you. Thoroughly enjoyed it.

  5. #5 OKThen
    April 11, 2012

    Of course this is really excellent science.

    “Understanding a supernova requires a good record of its rise and fall in brightness, or light curve. Although SN 2007bi was detected more than a week after its peak, Nugent delved into years of data compiled by NERSC from the SNfactory and other surveys. He found that the Catalina Sky Survey had recorded SN 2007bi before its peak brightness and could provide enough data to calculate the duration of the rising curve, an extraordinarily long 70 days — more evidence for the pair-instability identification.”
    Amazing work.

    “”It’s significant that the first unambiguous example of a pair-instability supernova was found in a dwarf galaxy,” says Nugent. “These are incredibly small, very dim galaxies that contain few elements heavier than hydrogen and helium, so they are models of the early Universe.”" and ..”Dwarf galaxies are ubiquitous but so faint and dim — “they take only a few pixels on a camera,” says Nugent, “and until recently, with the development of wide-field projects like the SNfactory, astronomers had wanted to fill the chip with galaxies” — that they’ve rarely been studied. SN 2007bi is expected to focus attention on what Gal-Yam and his collaborators call “fossil laboratories to study the early Universe.”" Which brings me to my question.

    Is there observational evidence that there were more dwarf galaxies 10 or 13 billion years ago than today? Or is “fossil” term applied to these galaxies due only to theory? I just want to know; because to me “fossil” observations (i.e. things observed 10 or 13 billion years ago) but not nearby (i.e. in the local group) are EXTRA IMPORTANT, to me anyway.

    I suspect the answer is that 3we 3don’t have enough observations to compare demographics of nearby versus far dwarf galaxies. But what is the status of th5is research?

    Thanks, for the summary of this excellent and very import research.

  6. #6 Spaceman Spiff
    April 11, 2012

    You said: “While nuclear fusion is occurring, the outward radiation pressure from the fusion in the core holds the rest of the star up against gravitational collapse. But when that nuclear fusion in the core runs out, the core collapses under its own gravity,…”.

    This is simply incorrect. As self-gravitating spheres of gas, stars maintain hydrostatic equilibrium by balancing thermal gas pressure against gravity (qualitatively, speaking). At sufficiently high T (for some value in gas density), radiation pressure also becomes an important contribution. But for most stars, radiation pressure is not at all important to the equation of state. Even in stars where radiation pressure is important, it is not “radiation pressure from nuclear fusion” supporting the star (or its core) against violent dynamical collapse. The ambient matter is hot and dense, and as such generates thermal gas pressure and emits a blackbody spectrum of photons with momenta (and so radiation pressure).

    What is the role of nuclear fusion in stars? In main sequence stars, for example, energy generated per second from hydrogen fusion in the star’s core merely allows the star to maintain energy balance (each Watt lost at the surface in the form of the star’s luminosity is balanced by each Watt generated from nuclear fusion in the core). In doing so, the star need not contract quasi-statically to maintain thermal pressure-gravity balance in the face of its energy losses at its surface (the star’s luminosity). Shutting down nuclear fusion would result in the star undergoing quasi-static gravitational contraction (over a thermal time scale of ~10 million years in a star like our Sun).

    Think of the formation of a star. A protostar first comes into existence as a self-gravitating sphere in hydrostatic balance, with energy generated via nuclear fusion being nil. It’s only when the proto-star has released sufficient energy via quasi-static gravitational contraction that it becomes sufficiently hot and dense in the core (given the star’s mass) to reach and maintain energy balance — and so arriving onto the main sequence.

    As for the pair production instability mechanism:

    From Scannapieco et al (The Astrophysical Journal, 633:1031–1041, 2005 November 10):

    “In stars above ~100 M_sun, after central helium burning, the core reaches a sufficiently high temperature and entropy to produce e-/e+ pairs, converting some of the internal energy of the gas into rest mass, which softens the equation of state to an adiabatic index below the critical value of 4/3 (Barkat et al. 1967; Bond et al. 1984). The result is a runaway collapse that leads to explosive burning in the carbon-oxygen core. For stars with initial masses more than about 140 M_sun, the energy released is sufficient to completely disrupt the star.”

    Why is an adiabatic index of 4/3 a critical value (i.e., P ~ density^{4/3})? Because by hydrostatic equilibrium the *required* pressure scales with density with the same power law index: P ~ density^{4/3}. So if the gas equation of state has pressure scaling with a *smaller power* in density, then the available pressure cannot meet the demands of the required pressure, and so the configuration becomes unstable to violent dynamical collapse.

    In the above passage, it explains that some of the thermal energy in the gas (contained within thermal gamma rays — the temperatures are above 1 billion K) is converted into “dead weight” e-/e+ pairs. This reduces the pressure support, forcing the core into a denser, hotter configuration which drives up the rate of pair production, reducing the pressure support,…., resulting in a dynamical collapse (time scales of ~seconds). The increase in temperature and density increases the energy released via oxygen burning, and a thermonuclear run-away thus ensues, catastrophically disrupting the entire star.

  7. #7 Wow
    April 12, 2012

    “This is simply incorrect. As self-gravitating spheres of gas, stars maintain hydrostatic equilibrium by balancing thermal gas pressure against gravity”

    This is simply incorrect. As an incandescent plasma, stars maintain hydrostatic equilibrium by balancing photon pressure against gravity.

    Failed stars like Jupiter and the massive Jupiters use hydrostatic pressure to maintain volume.

    If your proposition were true, how would stars turn into Red Giants? They COOL DOWN in the atmosphere!

  8. #8 Justicar
    April 12, 2012

    Wow, my understanding is that which is more important to a star depends on the star’s mass. The more massive stars are supported by radiation pressure, while the lighter stars (like ours) depend on thermal gas pressure to stay in equilibrium.

    Here, we’re talking about a massive star, so I suppose that radiation pressure is dominant.

    I’m not expert on stars though; they scare me – so I’d happily follow a discussion. =^_^=

  9. #9 Wow
    April 12, 2012

    “Wow, my understanding is that which is more important to a star depends on the star’s mass”

    That’s not my impression, nor of my lecturers when I did my degree in Physics with Astrophysics…
    :-)

    Not that they were always right, mind. One thought (though this may just have been to allow him to be dismissive and contemptuous of undergrads) that you whistled with your vocal chords.

    The photon pressure is immense in a star. It may well be that the outermost atmosphere (the least interesting bit to the existence of a star, but the most interesting to most astronomers and astrophysicists) is kept up with gas pressure, but the photon pressure is still pretty high even in the middle of the chromosphere.

  10. #10 Wow
    April 12, 2012

    PS Note: our star will turn into a Red Giant. It will, at the time, be slightly LESS massive than one solar mass then. But that would still have to keep itself up with “gas pressure” at a lower temperature.

    The core is a much HIGHER temp, mind, which is the source of all that photon pressure. And (IIRC) though the energy per fusion event is lower the higher you go up, and needs higher temps to get fusion started (hence a collapse needed), H-H fusion goes as T^12 or T^14. C-C fusion goes as T^16, O-O fusion as T^18 and so on. So at a certain temperature that can be attained, more energy is produced driving expansion to keep the rate of production at a level that keeps the atmosphere on the star.

    Of course this is a very delicate balancing act, therefore a minor change in density will make a huge change in temp and therefore a big change in size, which cools things down, and we overshoot the other way.

    Cepheid variables and other winky wonky stars.

  11. #11 Alex Besogonov
    April 12, 2012

    “Because a positron will run into an electron in short order, annihilating it, producing a gamma-ray. And if the rate of gamma-ray production is fast enough, you heat up the core. In other words, if you start producing these electron-positron pairs at a certain rate, but your core is collapsing, you’ll start producing them faster and faster… continuing to heat up the core!”

    That’s TOTALLY incorrect. You know, that pesky energy conservation law makes sure that the annihilation of a positron and electron produces exactly the same amount of energy as the originating gamma-photon.

    Instead, in pair-instability supernovae another mechanism takes place. During star’s NORMAL life (i.e. NOT during iron core collapse or anything) the energy of fusion in the core is enough to start producing electron-positron pairs.

    And that means DEATH!! Because it means that the effective pressure in the core suddenly _drops_.

    So the outer layers begin to fall down on the core, driving the temperature up.

    So the core begins collapsing. But unlike the inert iron core of regular core-collapse supernovae, the core of pair-instability supernovae still has plenty of fuel left (mostly helium, oxygen and carbon). So a significant part of this fuel burns in the space a FEW SECONDS in a giant stellar-sized thermonuclear explosion.

    Another interesting fact, pair-instability supernovae produce enough energy to totally unbind the star, literally blowing it apart. Core collapse supernovae can’t do this, because their explosion is driven by good old gravitational collapse.

    I.e. literally by a giant brick falling down a well – however you try, you can’t make the brick bounce of the bottom of the well and fly away.

  12. #12 Wow
    April 13, 2012

    “That’s TOTALLY incorrect. You know, that pesky energy conservation law makes sure that the annihilation of a positron and electron produces exactly the same amount of energy as the originating gamma-photon.”

    But since temperature is not merely energy, but a particular bulk expression of temperature, this isn’t the knock-down argument against the statement you thought.

    A 20Mev Gamma will create a profusion of e/e- pairs. Entropy and the temperature associated increases when you increase the number of entities.

    Yes, temperature is seriously wonky in a world where we understand energy…

  13. #13 Justicar
    April 14, 2012

    Wow, one thing I love about the internet is that I can read about how something works, and just as confidently be offered two opposite views. =P

    I’ve spent a few hours in the last two days desperately trying to avoid looking in the literature. And so far, I’ve managed to avoid that!

    Okay, so, I said that low mass stars like our sun depend more on gas thermal pressure than larger stars, which depend on radiation. You think I’m wrong (and I probably am).

    But, here my reasoning: the core is not the bulk of the star. In low mass stars like ours, you have a core that depends on radiation for energy exchange and transport, and outer layers that use convection.

    Larger mass stars have cores that are energetic enough to use convection for energy transport, and these stars’ outer layers will use radiation.

  14. #14 Justicar
    April 14, 2012

    Oh, Wow, if you’ll look up at comment I left up-thread about stars scaring me. Well, read SWAB’s newest article. I’m not particularly fond of studying all the different ways a stray and distant cosmic phenomenon could burn off Earth’s atmosphere, say as would happen with a high-energy gamma ray burst. And that’s just from stars qua star, the logically necessary precursor to even less friendly ways of the universe stamping us out: black holes.

    I’m glad there exist people who find this extremely fascinating and not creepy to study, for science requires such people. In the same way that there are many very fine scientists who would be lousy, lousy surgeons is how I am with cosmic phenomena. It’s fascinating work that is important to be done. And it creeps me out.

  15. #15 Alex Besogonov
    April 14, 2012

    Wow:

    Not really. Since e-/e+ pairs add more degrees of freedom your temperature is actually going _down_ because you increase your ‘heat capacity’.

    That’s what happens – effective pressure in the core goes down and it starts collapsing. If e-/e+ pairs would increase temperature then the core would simply expand until a new equilibrium is reached.

  16. #16 Wow
    April 16, 2012

    “Since e-/e+ pairs add more degrees of freedom your temperature is actually going _down_ because you increase your ‘heat capacity’.”

    That is also true, in the general case, but not the one here, Alex.

    “If e-/e+ pairs would increase temperature then the core would simply expand until a new equilibrium is reached.”

    But thereby reducing the e/e- pairs.

    As far as I can recall, this is what threw those people out who believed that the limit of an incandescent star was 60 solar masses, because the core would be beyond the Chandresekar limit and therefore turn into a black hole from the start.

    The star could slow its collapse and maintain a fusion core without collapse.

    Based on my interpretation of the maths I have read explaining this, with the proviso that my mathematical chops are in no way sufficient to understand it properly.

  17. #17 Wow
    April 16, 2012

    Plus (separated to stop it being held back), that expansion of the core is how you don’t get a (significant fraction of the mass) black hole here: the production doesn’t really need a density gradient of the same size as a fusion core to feed the photon pressure to keep the core from collapsing. Therefore the core isn’t as dense and so less or none of it is within the black hole event horizon when going boom.

  18. #18 jdmeth
    April 18, 2012

    As my mother used to say, “What does that have to do with the price of tea in China?” It seams to me that most of the highly intelligent people in the world spend their time debating how many angles can dance on the head of a pin. The only people tying to do something for the world are either wild-eyed activist agitating for social change or control freak politicians using the wild-eyed people to gain control of the population. Now if these observations lead to cheap fusion power then OK, carry on.

  19. #19 Wow
    April 18, 2012

    Since the head on a pin is fractal, there are a stupendous number of *angles* that are on it.

    None of them can dance, however.

    So, ignoring the typo, what, basically, does your post have to do with the price of tea in china?

    Nonstick frying pans were not what Shuttle engineers were looking to create.

    And, at base, this life is the only one we have.

    Why should they spend it doing only what YOU think is worthwhile?

    After all, no matter how much inventing of fusion power they do, they’re not going to get out of life alive.

    Carry on, people. Enjoy this life and if that enjoyment is taken in discovering this universe we live in, have at it, hoss.

  20. #20 Hannes
    April 19, 2012

    @Alex Besogonov

    I mostly agree with you here. Impressive comment!

    Since E=mc^2 the change from bosons to e-/e+ pairs will at first have a cooling effect on the core since converting some of the internal energy of the gas into rest mass will drop the pressure by “blackbody radiation”, which is actually the same as convection.

    Since the Second Law of thermodynamics states heat (“photons”) can only be transferred from hot_to_cold, the problem is that the e-/e+ pairs can only be created when there is a flow from hot_to_cold.

    Within the core there is most likely not enough difference in temperature to make that thermodynamically possible. Hot_to_hot creation of e-/e+ pairs is not possible, since it would violate the Second Law here.

    So in my view the e-/e+ pairs are only created when there is a big enough difference in temperature.
    Which would mean that the e-/e+ pairs will not be created in the core itself, but only outside of it.

    (Gammarays are detected on Earth because a part of it is NOT converted into e-/e+ pairs during the trip from coming_into _existence[in the sun] to creating muon showers[on Earth].)

  21. #21 Hannes
    April 20, 2012

    Btw, the situation I mentioned above is only relevant for the very first popping_into_existence e-/e+ pairs. After those are created the form a nearly perfect hot_to_COLD SITUATION.

    Before the ultracold e-/e+ pairs even share their “heat” with other particles by convection they are hit by other gamma rays creating an avalanche of coldness in the star.

    Imagine what zero Kelvin or minus 273 degrees Celsius particles do in the middle of an ultrahot star!

    I forgot to mention Spaceman Spiff, sorry

  22. #22 Kevin (NYC)
    April 22, 2012

    “Since the Second Law of thermodynamics states heat (“photons”) can only be transferred from hot_to_cold, the problem is that the e-/e+ pairs can only be created when there is a flow from hot_to_cold.”

    I was just reading up on this at the Science of Doom re GCC. The 2LoT does not apply to single photons but only to something that has a temperature. A temp is only defined for a collection of items using Statistica Thermodynamics.

    so photons can go anywhere they want, and can add energy to a particle at a higher level of energy if it interacts just right.

    don’t know if that changes what you were saying.. but..

  23. #23 Hannes
    April 23, 2012

    Thanks Kevin for your reply.

    2LoT could even be called 4LoT, since there is also a Zeroeth Law and a Third Law.

    The temperature of a single photon can indeed not be measured, true.
    However, to create or measure a single photon you need at least one particle emitting it – and also an observer watching. So there is always a physical interaction between two objects.

    I have to disagree partly with your remark that lower energy photons can add energy to a particle of higher energy. I had some discussion with user “WoW” about this, in an earlier thread. The problem is that if a flow from cold_to_hot is possible, it would also be easy to produce “Work” in general.

    For instance, in an isolated room with the same uniform temperature (hot_to_hot situation), you could place a one-way mirror in the middle. If photons would be present, part of them would be reflected to a single side. One side would heat up, the other side would lose energy. Creating a temperature difference.

    However, this is not possible in reality. What makes it all complicated in astrophysics is that when observing a star far away, we observe it as having a “low” energy output.
    Because of the distance it is measured as a “low” energy photon. So SEEMINGLY coming from a lower temperature object. Which is not the case.
    It will heat up the observer, only by a very small amount- yes. So the math works, but does not explain the circumstances.

    The reason energy only flows from hot_to_cold is [in my view] that photons are only “real” if they are measured.

    But maybe you are asking why those e-/e+ pairs are for a very brief time so very cold (0 K). This is because E=mc^2 would be flawed if there was any additional energy available at the creation of those e-/e+ pairs. They have to be very cool. But only for a very brief amount of time, of course. They will annihilate very quickly.
    But light is quicker than convection, the harm to the star will been done in a flash of a second. Energy has been converted to mass, reducing thermal pressure.

  24. #24 Hannes
    April 23, 2012

    Before having a discussion with “WoW” or someone else again:

    Yes, lower energy photons will definitely add energy to a particle of higher energy.

  25. #25 Kevin NYC
    April 23, 2012

    hey I understood a comment and got something right!

  26. #26 Wow
    April 26, 2012

    “The problem is that if a flow from cold_to_hot is possible, it would also be easy to produce “Work” in general.”

    The problem is that you are deliberately getting it wrong.

    NET FLOW is what the second law of thermodynamics is about.

    But you really don’t want to accept that, do you: it’s inconvenient to your faith (in the Free Market).

  27. #27 Wow
    April 26, 2012

    “since converting some of the internal energy of the gas into rest mass will drop the pressure by “blackbody radiation”"

    Except that photons have mass and, to be at any temperature above absolute zero, the particles aren’t at rest either, what do you think you’re doing by making the fake distinction about rest mass for things that ARE NOT AT REST?

  28. #28 Hannes
    April 27, 2012

    Photons only have momentum, not mass.

    E^2 = m^2c^4 + p^2c^2

    With m=0 [rest mass of a photon] you get E = pc or p = E/c.

    For a particle at rest [p=0] you get E=mc^2.

    If somebody would be able to get a flow of energy from cold_to_hot he/she would be a very rich man.

  29. #29 Hannes
    April 27, 2012

    I don’t know if they have an official name for it, but I would call the process an adiabatic cooling of a star.

    Once the first preliminary conditions are set, determined by the thermodynamic energy_density value [threshold 2 times 511,007 keV = 2 times 9,109534×10^-31 kg] to trigger the event of an electron/positron pair creation, there is no stopping of this avalanche_of_creation. It will spread with the speed of light throughout the star. Wherever it started btw.

    Since E=mc^2, the pairs that have been created will have zero momentum [p=0] from the beginning of their creation.

    Which implies they have no relative speed to other nearby particles when they are formed.

  30. #30 leniya
    oregon
    April 3, 2013

    wow cool

  31. #31 Wow
    April 4, 2013

    “I don’t know if they have an official name for it, but I would call the process an adiabatic cooling of a star.”

    adiabatic: An adiabatic process is any process occurring without gain or loss of heat within a system (i.e. during the process the system is thermodynamically isolated)

    “Photons only have momentum, not mass. ”

    And momentum requires mass.

    “With m=0 [rest mass of a photon] you get E = pc or p = E/c.”

    A photon going at zero speed doesn’t exist as a photon. Therefore its rest mass is irrelevant: it always has its relativistic mass.

    “Since E=mc^2, the pairs that have been created will have zero momentum [p=0] from the beginning of their creation.”

    No, the vector sum of the pairs momentum is zero, but both have momentum.

    Don’t talk such crap.