Sunday Chess Problem

I had originally picked out another Circe problem for your enjoyment this week. However, this particular problem was in the genre of “series” movers, and it occurred to me that I had never shown you one of those before. I didn't want your first one to be the rather complex affair I had selected. So, instead I picked out a simpler series problem, just to illustrate how the genre works. But after preparing this problem, I then discovered that the Circe series problem I wanted to use turned out to be cooked. Oh well. You're getting this one anyway!

This problem was composed by George Sphicas in 1992. The stipulation calls for Series Selfmate in 14:

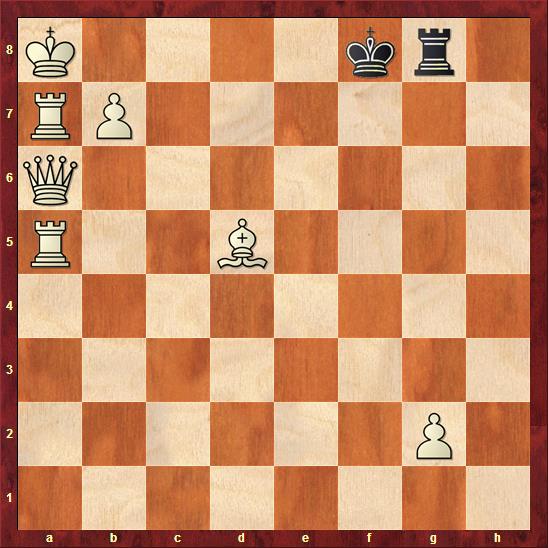
The idea is this: White is going to play fourteen consecutive moves. During that time, black will remain motionless. After white's fourteenth move, the position on the board will be such that all of black's legal moves will result in white being checkmated. (That's what makes this a series selfmate, as opposed to some other genre like a series helpmate). During his series white is allowed to capture all the black pieces he wants. The only restriction is that he must not put black in check, with the possible exception of the last move.

For a series mover to be considered sound, it must be that the moves of the series have to come in an exact order. If even two moves can be switched, then the problem must be discarded as cooked. Part of the fun of series movers is working out the reasons why the moves must come in the order they do.

So let us see what we have. Black is only going to make one move. We must envision what the final position could possibly be that will compel that move to be checkmate. Since the white king is already cornered, you might think the idea is to cover all of his flights, and then compel the black king to move from the back rank, thereby discovering check from the black rook.

Alas, if you spend some time trying to make that work you will find it is a no go. White must work harder. Let's have a look. The solution begins with 1. Ra2 2. Re2:

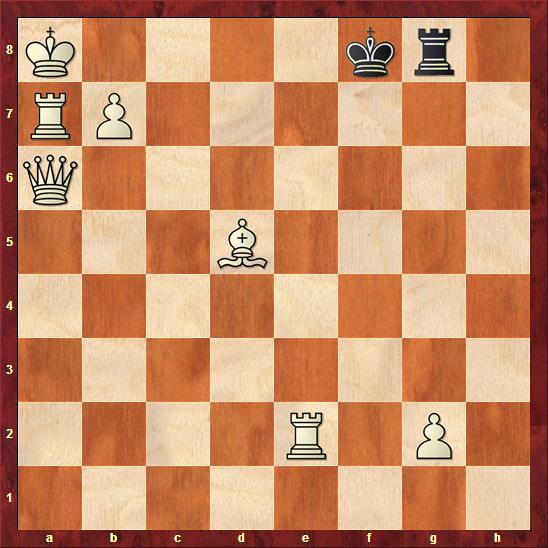
The white rook has cleared a line for the white queen, and it will eventually prevent the black king from moving to e7 or e8. But why couldn't the rook have achieved those ends by moving to e4, e3 or e1 instead? Stay tuned!

The white queen now makes use of her new-found freedom to play the dramatic 3. Qa1 4. Qa8 5. Ra1:

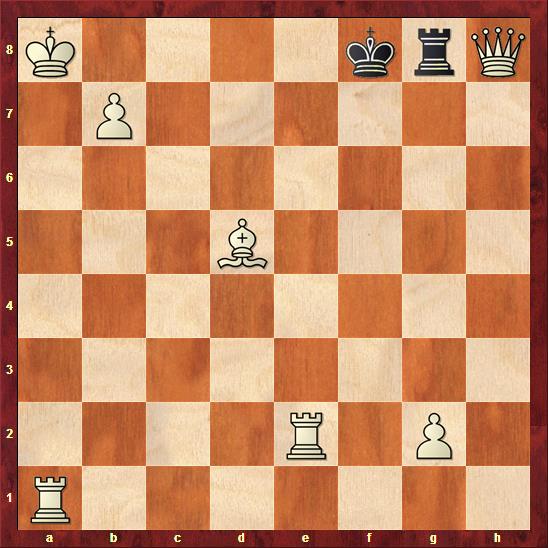
The white queen goes corner to corner, pinning the black rook. The queen had to use this eccentric route, since the only alternatives go through f6 or h6, which would have checked the black king.

As for the Ra1 move, wait and see! We now come to the main point of the problem. As if to complement the white queen's trek, the white king will also move corner to corner. Since the white king must not cross the g-file, because of the check from the black rook, there is a unique, shortest route for the king to make his trip: 6. Ka7 7. Kb6 8. Kc5:

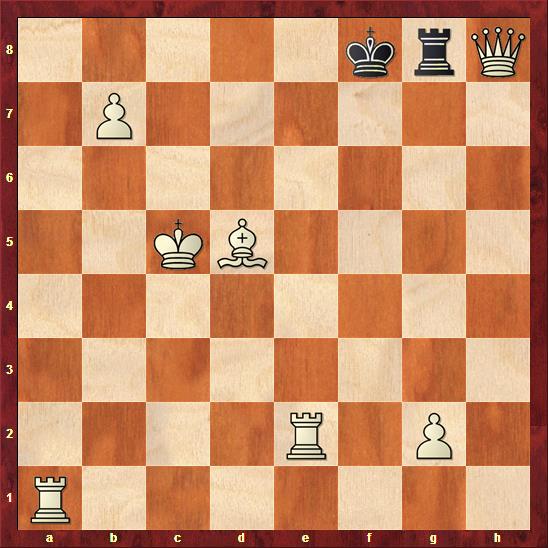
Get the idea? We continue: 9. Kd4 10. Ke3 11. Kf2:

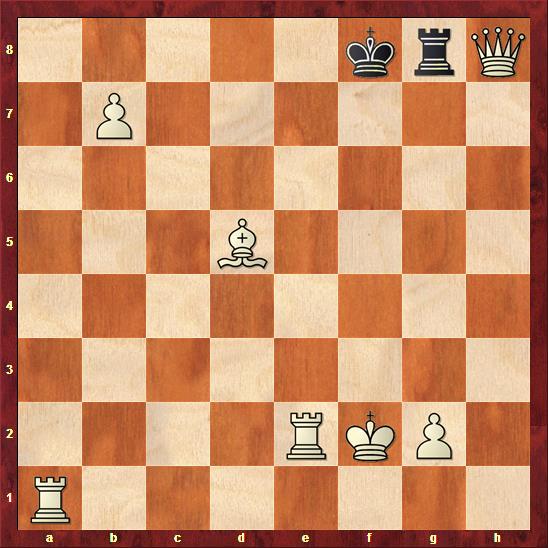
And now for the dramatic finale. White plays 12. Kg1 13. Kh1 14. Rg1, creating this position:

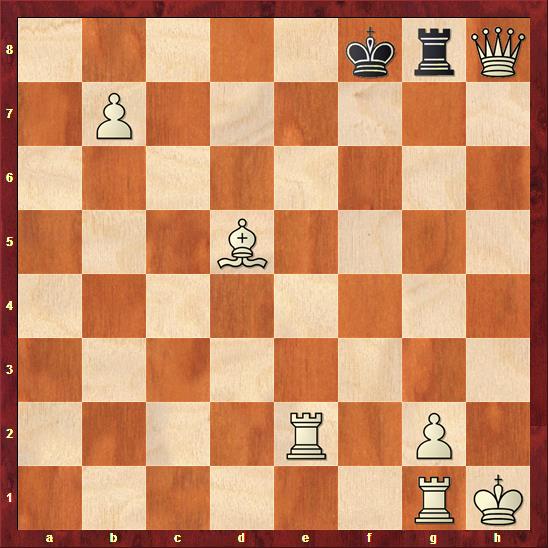
Black finally gets to move, but he only has one option, which is to play 14. ... Rxh8 mate.

Now we see why the first white rook had to choose its destination square so carefully. From e4 or e3, the rook would have interposed on the h-file. From e1 it would have interfered with the other rook's route to g1.

Very pretty stuff! See you next week.