The Physics of Crazy Sleds

In the Uncertain Dots hangout the other day, Rhett and I went off on a tangent about the physics of the Olympics, specifically the luge. If you're not familiar with this, it's basically psycho sledding: people riding tiny little sleds down a curved track at 80mph.

The "featured image" above shows Erin Hamlin of the US women's luge team during a training run (AP photo from here); she went on to win a bronze medal, the US's first in individual luge, so congratulations to her. The photo gives you an idea of what's involved: tiny sled, icy track, curved walls.

(In the Winter Olympics context, this actually seems relatively sane, because there's another sport, called "skeleton," which is basically the same thing, only face-first. This is basically the same progression followed by kids at the local sledding hill, so I expect in another dozen years or so, they'll make the skeletoneers look rational by introducing a sport where lunatics ride down the track backwards, or standing on the sled like a pseudo-snowboard.)

Anyway, Rhett mentioned that he'd gotten interested in this when his daughter asked why there was any difference at all in the times for this event, given that they're all riding the same sleds down the same track. That's an interesting point, and provides a decent starting point for thinking about the physics of the luge.

By a weird coincidence, I had also been paying attention to the luge, because one of the US men's team, Tucker West, is going to be starting college at Union in the Spring term, once he gets back from the Olympics. Our PR office was bragging about this on Twitter, and I started following West's feed (he finished 22nd, for the record, which might seem disappointing, but ask yourself: are you the 22nd best in the world at anything? Were you at age 18?). What made me start wondering about this was that in various retweeted photos of West and other lugers-- this one, for example-- they're bigger than I expected. For some reason I assumed they would be smaller, probably because of the little sleds, but if you look at the US team, they're typically around six feet, 180lbs, which isn't gigantic (keep in mind, I'm 6'6", 280lbs, so my perspective may be skewed), but is a clearly bigger than average. So, I started wondering if there's a physics reason why size would be advantageous in this.

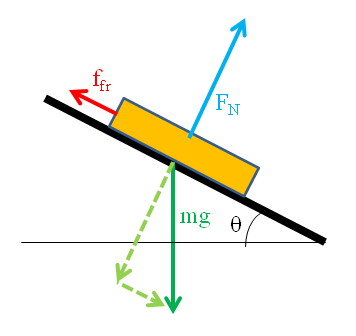
That might seem like a stupid question, given that bigger athletes are, by definition, pulled downhill more strongly by gravity, but in fact, when you think carefully about the physics, it shouldn't matter. If you look closely at the forces acting on an object sliding downhill, you see something like this:

Diagram showing the forces on an object sliding down a slope.

The vertical green arrow is the force of gravity, which by definition is straight down. The up-and-to-the-right arrow is a "normal force" from the surface, "normal" here being a mathematical term of art meaning "perpendicular to the surface." And the red arrow pointing uphill is a frictional force resisting the slide-- which is present even if you're sliding down an icy track on a ridiculously small sled.

These three forces add together to give you the net force acting, but they're not completely independent of one another. The normal force is however big it needs to be to keep the object from sinking into the surface, which is determined by the force of gravity. The frictional force, in turn, depends on the normal force-- the harder the object is pressed into the surface, the bigger the force of friction.

When you work it out, then, all of the forces are related to the mass and the strength of gravity. When you look at how quickly the object moves down the slope, what matters is the net force along the slope, and when you work it all out, that comes out to:

$latex F_{net} = mg(\sin \theta - \mu \cos \theta ) $

(where $latex \mu $ is a factor that accounts for the dependence of friction on the normal force) That depends on the mass, but the speed is ultimately determined by the acceleration of the mass, which you get from Newton's second law, $latex F_{net} = m a $. And that also has a mass in it, on the other side of the equals sign from the force, so when you solve for the acceleration, you find:

$latex a = g(\sin \theta - \mu \cos \theta) $

So the acceleration is the same, regardless of mass. This is why, even though I outweigh them by nearly an order of magnitude, I don't go down a playground slide any faster than my kids, as you can see in this video that I use in intro mechanics classes:

Thus, a simple physics argument would suggest that being heavier isn't actually any benefit in downhill sliding events.

But, as I said in the video, I started wondering about whether there might be some benefit when it comes to going around corners. As you can see from the photo above and the point-of-view video in this feature from the New York Times, the track involves banked curves, and the sleds ride up the wall as they go around the curves. That's a somewhat more complicated situation, so maybe there's a benefit to being big from that-- maybe really light riders will end up going way up the wall, and thus take a longer path, leading to a slower time.

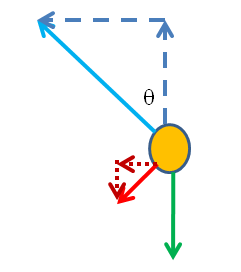
The physics in thinking about the banked curves looks like this, where I've kept the same color scheme as the previous picture:

A view of a object on a banked curve, showing the forces that act.

You can imagine this as a view down the track, from an utter lunatic following the sled down the hill. Again, there's a force of gravity straight down, and a normal force that's perpendicular to the surface, up and to the left. There's also a frictional force, in this case down and to the left, keeping the sled from sliding perpendicular to the track.

Again, the behavior here is determined by adding all these forces together. In this case, though, we know an additional piece of information, namely that the sled is going around a curve with some radius R (the center of which would be off to the left somewhere). According to Newton's laws, though, the sled really wants to move in a straight line, so there must be some net force acting to bend it onto this curve, and that "centripetal force" is:

$latex F_{cent} = m \frac{v^2}{R} $

Where v is the speed, and R the radius of the curve-- so higher speeds require bigger force, as do tighter curves (smaller R).

So, all the forces in that picture need to add together to make this centripetal force, or else the sled will bust through the track and continue in a straight line. Which would be Bad. If we want to see how this works out, we need to break these forces up into components that point straight up-down and left-right, and recognize that the centripetal force comes only from the leftward part.

A closer look at the forces on the banked curve, showing the components

The normal force has an upward piece and a leftward piece, and the frictional force has a leftward piece and a downward piece. Those leftward bits are what we really care about, and they're related to the total forces by simple trigonometry.

This might seem like a hopeless problem, though, as the normal force is whatever it needs to be to keep the sled from breaking through the wall, up to the strength of the materials involved. And the friction force is whatever it needs to be to keep the sled from sliding across the track. Which means we've got more variables floating around than we can easily deal with.

But, again, these things are related to each other in a way that lets us set a limit. That is, there's a maximum possible value for the frictional force, that's proportional to the size of the normal force. And the normal force needs to counter the downward pull of gravity, so we can set the size of the upward component, and with a bit of trigonometry relate everything to the angle between a vertical line and a line from the center of the curved track to the position of the sled. The algebra gets a little gory, but in the end, you find a net horizontal force that looks like:

$latex F_{net} = mg\frac{\sin \theta + \mu \cos \theta}{\cos \theta - \mu \sin \theta} $

That might seem a little scary, but we can do some simple checks to see if it makes sense. If the angle is zero, so the sled is flat on the bottom, then the force is just the frictional coefficient multiplied by the weight, as it should be-- the normal force can't contribute to a horizontal centripetal force, so it's all just friction. As the sled goes up the wall, the net force gets bigger, because the normal force starts to include a horizontal component, which is why they bank the curves in the first place.

So, when you go through all this, what do you get? Well, that net force depends on the mass, but when you set it equal to the centripetal force, the mass drops out again, just as it did in the initial problem. Light or heavy, it makes no difference-- the angle and the coefficient of friction are the only things that matter. You can find a maximum speed for a given angle, which is given by:

$latex v_{max}^2 = R g\frac{\sin \theta + \mu \cos \theta}{\cos \theta - \mu \sin \theta} $

Any faster than that, and the sled won't have enough inward force to stay on the curve at that angle, and will slide outward.

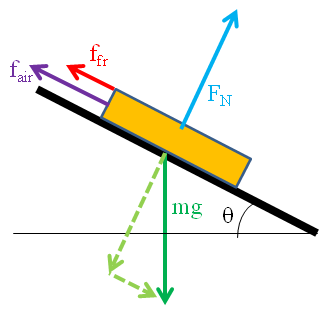
So, why are these guys on the large side? In the end, Rhett probably has the right answer in the hangout video: it's all about air resistance. When they're sliding down the track, the picture up above needs an extra force arrow:

The sliding object again, this time with air resistance.

The air resistance force is utterly insignificant for slow objects like me and SteelyKid going down playground slides, but when you're rocketing down an icy chute at 80mph, it's very significant-- the force generally goes like the square of the velocity. It doesn't depend on the mass, though, so the downhill acceleration becomes:

$latex a = g(\sin \theta - \mu \cos \theta) - \frac{f_{air}}{m} $

That second term tends to reduce the speed, but the effect is smaller for heavier masses. So, being somewhat bigger is an advantage, at least to a point-- the air resistance force will depend on the cross-sectional area of the sliding object, so a big fat guy isn't going to do very well, because he'll have to push a lot of air out of the way. Taller guys add mass without increasing the cross section all that much, though; thus, the initial photos that surprised me of luge team members towering over NBC reporters.

Of course, that doesn't entirely answer Rhett's daughter's question as to why there are differences in the times. After all, this would suggest that two lugers with the same mass and cross-sectional area should have exactly the same time, every time. But of course, there's more to it than that, and I have a math-y explanation of (part of) that, as well. But this is getting long as it is, so I'll stop here, and talk about steering in a later post.

------

(One might object that the coefficient-times-normal force model doesn't really work for the case of a sled with blades biting into ice, but I think you can still model it that way, just with a much larger $latex \mu $ than for smooth objects sliding over each other. Which explains Rhett's results in his speed skating post.)