Mr. Harley: Your impatience is quite understandable.
Klaatu: I'm impatient with stupidity. My people have learned to live without it.
Mr. Harley: I'm afraid my people haven't. I'm very sorry... I wish it were otherwise.
-The Day The Earth Stood Still, 1951
People love to talk about the constancy of the natural phenomena. Every 24 hours, from anywhere not within either the Arctic or Antarctic circles, for instance, there's one sunrise and one sunset.
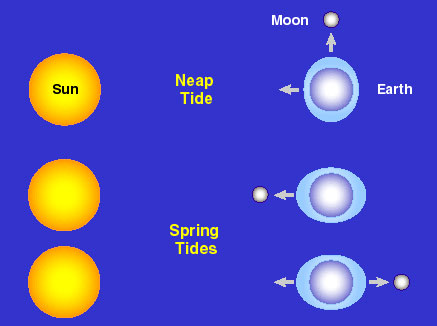
And, of course, there are two high tides and two low tides every day.
The Earth rotates on its axis, and revolves around the Sun. And this, pretty much, is the way things are, and always were, and always will be.
Well, kind of.
What if, instead of spinning a sphere in space, like the Earth, you were to spin a top, but in a completely frictionless environment?

It would continue spinning forever, like in that movie.
But what if I put just a tiny little force on it? Just enough, maybe, to add a little bit of friction? Even the air is sufficient to do it.
Well, of course, in the case of a top, it will lose speed and fall over. But the big deal for me is this: it slows down! So if something exerts the right force on the Earth, perhaps it will slow down, too. Any candidates?
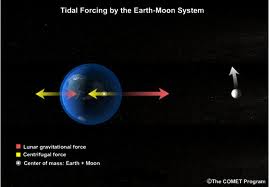
The Moon! The Moon -- gravitationally -- exerts a greater force on the side of the Earth closer to it than the side farther away.
This differential force results in a tidal acceleration that slowly pushes the Moon farther away, and also very, very slightly slows the Earth down!
Think about it a minute. Every day contains 24 hours, which in turn contain 60 minutes, which in turn contain 60 seconds apiece, for a total of 86,400 seconds in a day. But if the Earth's rotation is slowing down, that would mean that in the future, we'll need more seconds to add up to a day, and that we needed fewer in the past.
How can we test this?

Look at tidal rhythmites, or the daily patterns left by the changes in the tides! Amazingly, we find patterns left behind from 620 million years ago, and find that a day back then lasted under 22 hours!
In fact, the International Earth Rotation Service confirms that the day gets longer by 1.4 milliseconds every century. And while that may not seem like much, it really adds up over billions of years.
If you extrapolate back to when the Earth was first formed, you find that a day was only around 23,000 seconds, or six-and-a-half hours! And it continues to slow down! Every 18 months or so, because of the difference between 86,400 seconds and an actual day, we add an extra leap second to our clocks.
As we continue into the future, the day will continue to slow down, there will eventually be fewer days in a year, and after another 4 million years or so, we can totally eliminate the need for leap years!
So although the laws of physics are solid, the communication between the Earth and Moon changes enough that the tides, the days, and even the years are altered over time. And we've been around long enough that this really matters! Hope you learned something new, because it's really amazing that we can measure time and changes this precisely, and find that the Earth is really slowing down!







how can no one comment?! wonderful info for my students!
The IERS curve shows the length of day decreasing over time, on a decadal scale :) Nevertheless, in the long term THA MOON wins ;)
wereatheist
Correction: "in the long run" (I'm no anglophonic native)
It seems to me that if the day is getting longer by 1.4 milliseconds/century then that doesn't account for a leap second every 18 months or so. What am I missing?
Dear Mr Siegel,
I generally only understand 1/4 of your posts, but I do purely love them. This is another good'n. Thank you
Cool stuff! I wish the Bill O'Reillys of this world would take some time to read about celestial mechanics. It's too bad I won't live long enough to enjoy 26 or 27 hour days, I always thought the days were too short, not enough time to do all the things I want to do and get enough sleep.
And the whole thing is evolving toward a system where the earth always keeps the same face toward the moon, so a day and a month are the same length and you can only see the moon from one hemisphere.
Richard: Remember that *every day* gets longer by 1.4 milliseconds per century. Multiply that by 365 days and it very quickly becomes noticeable clock drift. The leap second every 18 months is because we still use the standard of 86400 seconds in a day but this has been drifting away from that perfectly round value for a while now. Rather than keep changing the definition of how many seconds are a day, we slip in an extra when no-one's looking and call it even.
At least, I think that is what is going on. :)
@Richard
Leap seconds is not used to compensate for the last 18 months of slow-down, it is used to compensate for the difference between a day as it is defined by SI and the current length of a day.
from Wikipedia:
The leap second adjustment (which is approximately 0.6 seconds per year) is necessary because of the difference between the length of the SI day (based on the mean solar day between 1750 and 1892) and the length of the current mean solar day (which is about 0.002 seconds longer). The difference between these two will increase with time, but only by 0.0017 seconds per century. In other words, the adjustment is required because we have decoupled the definition of the second from the current rotational period of the Earth. The actual rotational period varies due to unpredictable factors such as the motion of mass within Earth, and has to be observed rather than computed.
Maybe this is obvious, but there's something I don't really understand:
How come the moon is moving further away, as opposed to falling towards the earth? When an artificial satellite loses energy it falls towards the earth, so how is the moon different? Is it somehow converting the rotational energy of the earth into potential energy?
What I would like to know is how much effect will there be if renewable energies, that are powered by the earth's rotation (such as wind and I suppose wave energy too) are used to a far greater extent. Will that make the slowing of the rotation worse?
@10 Emlyn
I believe (and I'm no physicist) it's because the moon collided with Earth, rather then being a product of the spinning gasses and dust, already trapped by gravity, that would have been orbiting the original body(ie how the planets were formed around the sun). Since it smacked into us, loosing the majority of it's potential energy, but not all, it's slowly been moving away. And in the distant past, it would have looked a lot bigger in the sky! And in the distant future, it will look a lot further.
Sooo... Bill O'Reilly is trying to hide the decline in the earth's rotational speed?
@Emlyn- this sounds like simple conservation of energy to me, if the force exerted moon is reducing the rotational kinetic energy of the earth, the moon should gain energy.
... force exerted *by the* moon....
BaisBlackfingers - yes, but there is also dissipation of energy within the Earth. What's being conserved in the motions (leaving out the effects of the Sun's gravity) is angular momentum.
But, yes, the Moon gets some orbital energy from the Earth's rotation.
iqhira - that's a brilliant question! I think the answer's yes, at least for tidal power (I think wind is mostly sunlight-driven); my intuition is that the effect is minute compared even to the 1.4ms/century, but it would be nice to know.
Thanks for the answers, I think I understand now: the difference is that artificial satellites are slowed down by friction, which causes them to fall towards the earth, while the moon is slowing the earth's rotation with tidal forces, causing it to move further away due to conservation of angular momentum.
So does this mean that once the tidal forces have stopped the earth rotating relative to the moon, the moon will stop moving further away? In which case could we calculate how far the moon will eventually get, just by conservation of angular momentum? i.e. at what distance would the moon have an angular momentum equal to it's current angular momentum plus that of the earth's rotation.
"The Moon! The Moon -- gravitationally -- exerts a greater force on the side of the Earth closer to it than the side farther away.
...
This differential force results in a tidal acceleration that slowly pushes the Moon farther away, and also very, very slightly slows the Earth down!"
I believe you have this wrong.
The force generates no torque on the rotating Earth-Moon system so cannot accelerate the moon away.
What happens (IF I am remembering my celestial mechanics correctly) is that the tides of the earth are offset from that straight line (from the POV of the Moon which is a few light seconds away) and that this offset causes a torque on the system, since the lump preceding (and this is where I KNOW I can't remember it: is the nearer lump preceding or lagging the moon rotation?) will pull the moon along the Moon's tangential vector, it speeds the Moon up. The reaction to that is to slow the rotation of the tide around the earth and slow the Earth down.
This WAS many years ago I learned this or something vaguely similar.
"It seems to me that if the day is getting longer by 1.4 milliseconds/century then that doesn't account for a leap second every 18 months or so. What am I missing?"
As well as the elements others here have supplied, the other planets perturb the orbit of the planet earth unpredictably (google for "the three body problem" and chaos which was the answer to the question regarding earth's perpetual orbit in the 17th(?) Century). That means that to get the Earth back in the same place (wrt the distant stars which don't move appreciably even for our exacting timing needs) takes a slightly different number of invariant seconds every time we go round the sun.
Therefore the number and location of leap seconds varies somewhat unpredictably.
Rocket science may not be rocket science (it's really rather easy), but orbital mechanics really IS rocket science!
"But, yes, the Moon gets some orbital energy from the Earth's rotation."
See also "the slingshot effect". The satellites we sent to Neptune via a slingshot from Mercury slowed mercury's orbit down to get there.
Orbital mechanics again.
:-)
Just to further complicate things, there are places that have one high tide, and one low tide, per day (for the value of "day" in terms of tides, which is slightly over 24 hours). This has to do with the shapes of certain landforms and bodies of water.
This doesn't affect the conservation of angular momentum and day length stuff—the changes there will be because of another slow process, plate tectonics.
Wouldn't this also mean that since the earth was spinning faster there was also more gravitational force. So objects would have been heavier towards earths beginning and will be lighter in the future?
Wow @17,
That is the other part of the dominant effect; good for you for nailing it!
Take a look at this image: http://en.wikipedia.org/wiki/File:Tidal_braking.svg
Any part of the Earth that bulges not along the line-of-sight to the Moon will allow the differential force to exert a torque, and the tidal bulge is the greatest contributor to that. Thanks for going above and beyond!
Hopefully my house is sitting on the side that will be facing the sun- gotta think about my property values in the future.
But I thought the first 7 'days' were billions of years long. Well, there goes old earth creationism.
But I thought the first 7 'days' were billions of years long. Well, there goes old earth creationism.
Yay! The creationists are crushed! *Snerk* *snerk*!!!111!!
So this is why my circadian rhythm keeps getting messed up. I knew it had to be something!
I wonder how it has been reconstructed that when the Earth was just formed the day had a duration of about 6.5 hours.
If I remember correctly the problem is as follows. When the land masses of the Earth are all spread out the fact that the Earth's water is separated in basins reduces the response to the tidal forces from Moon and Sun.
Currently the Antarctic sea is the only body of water where the heave of the tide can actually circumnavigate the Earths axis. It is my understanding that the tidal movement of the Atlantic ocean is secondary, in the sense that is is a wave of tide that originated in the antarctic sea. So for, say, Europe, each tide surge it experiences is a wave that took something like a day and a half to travel north from the antarctic sea.
Over hundreds of millions of years Earth land masses come together to form a single supercontinent, and then drift apart again. There have been several of those cycles. When there is just a single supercontinent the Earth's water is much more free to respond to tidal forces. It is my understanding that when there is a single supercontinent tidal braking is stronger than when the Earth water is divided into separate basins.
To my knowledge that is why it's very tricky to try and reconstruct the earliest Earth rotation rate. The tidal braking rate is not uniform.
You can't explain that!
This is nice to know but unfortunately our mind's perception of time's passing moves faster as we get older which more than cancels out the extra time each day we get from our moon dance (joke).
Related somewhat: Any thoughts on whether the more powerful ancient lunar tidal forces might have enabled dinosaurs to grow so large when simple mechanics would suggest it is doubtful whether such large land based creatures could exist today? Move at high tide, sleep at low? I sometimes feel like that after a big meal.
If the Earth is slowing in its rotation would the movement of the magma around the iron core also be slowed reducing the magnetic energy at the poles leaving Earth more vulnerable to solar flares?
It seems to me that if the day is getting longer