"This is to show the world that I can paint like Titian: [See drawing, above.] Only technical details are missing." -Wolfgang Pauli
First theorized by Pauli all the way back in 1930, neutrinos are some of the most mysterious and puzzling particles ever discovered in nature. For starters, they weren't even first detected until 1956 (by Reines and Cowan), 26 years after they were predicted to exist! Coming in three flavors -- electron, muon and tau -- and in both particle and anti-particle type, these neutrinos have the smallest but non-zero masses of any particle ever discovered.
Image credit: Argonne National Labs.
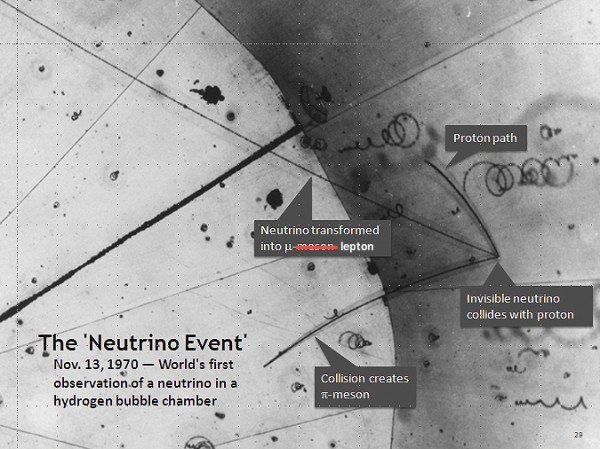
The history of these elusive particles is literally a treasure trove of riches into the fundamental nature of our physical world. The picture above -- the very first of a neutrino in a hydrogen bubble chamber -- is a fantastic example. Over to the right of the image, you can see what looks like a bunch of tracks all originating at some vertex.
This image -- generated in 1970 -- is a surefire sign of a neutrino striking a proton, something we can definitively tell by the tracks of the particles coming off. (None of which, by the way, is a neutrino!)
Image credit: Argonne again, retrieved from Universe Today, and corrected by me.
Based on the curvature and radii of the tracks left by the particles that come out of this interaction, since they're all in a known magnetic field, we can determine their masses, charges, and velocities, and hence we can reconstruct that this was a muon neutrino that struck a proton, producing a negatively-charged muon (to conserve lepton and lepton family number), an oppositely-charged pion (to conserve electric charge) and kicking the proton off (to the upper right)! You can tell the charge of any particular particle by the direction it curves in the magnetic field: positive ones curve counterclockwise, negative ones curve clockwise!
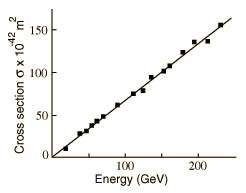
Neutrinos are incredibly difficult to detect: we need to literally make quadrillions of them at the highest energies achievable just to have a reasonable chance at detecting one neutrino, and the lower in energy your neutrinos are, the more steeply their cross-section drops, as the graph below shows.
Image credit: J.W. Rohlf, retrieved from hyperphysics.phy-astr.gsu.edu.
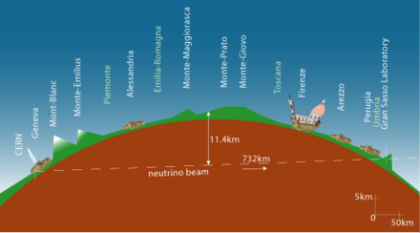
And, as you all know, recently a beam of very high-energy neutrinos was sent from CERN to Gran Sasso. Something remarkable like 1020 neutrinos were generated and launched towards the OPERA detector, hundreds of miles away beneath an Italian mountain, and a few thousand of them were detected. Oddly enough, on average, they were detected about 60 nanoseconds too early, resulting in rampant speculation that these neutrinos were traveling faster than the speed of light!
Image credit: CERN neutrinos to Gran Sasso.
I wrote a large number of articles about this, and even went on TV to talk about it. Most recently, I was invited to be a guest href="http://scienceblogs.com/startswithabang/2011/11/weekend_diversion_neutr…">on the radio to talk about these fascinating particles (episode available here), and as is often the case, didn't get a chance to answer everyone's questions.
Well, the skeptics over at Skeptically Speaking were kind enough to send me the unanswered questions, and I'm pleased to take them all on here for you! Let's get to it...
Image credit: Boeing, retrieved from Inside GNSS.
1.) Early on there was an initial response that this result had been explained because of a measuring error involving special relativity and GPS. What was this criticism and do we know yet if the OPERA team had accounted for this during their initial experiments?
It's better to start by reminding you what a GPS satellite is: it's basically a satellite in space a known distance away from the surface of the Earth, with an atomic clock on board. You take an atomic clock at your location on Earth, and you can tell what the light-travel-time (and hence the distance) from your location to the satellite is. By taking a number of GPS satellites (usually four), you can precisely know your position and the amount of time that's elapsed from any particular event on Earth. But you need to be smart about it: both the effects of special relativity (how quickly the satellites and you, on Earth, are moving as the Earth rotates on its axis and revolves around the Sun) and general relativity (the gravitational effects of the Solar System) must be taken into account! If such effects weren't taken into account, a GPS satellite could botch your position by as much as 30 meters.
How long does it take light to travel 30 meters? 100 nanoseconds. So it's conceivable that this systematic, 60 nanosecond shift is entirely due to a GPS error. However, this is well-known physics, so it's pretty reasonable to assume that the OPERA scientists know how to account for this. (Quite honestly, it would be shocking if this were where the error lied.) But, as they didn't release this information publicly, the only way to check is to have a different team do the experiment over.
Image credit: Jbourjai on wikipedia.
2.) Are the extra dimensions theorized as part of string theory a possible part of the explanation for these neutrinos?
Although this is one of people's favorite areas of speculation, the answer is "probably not." Sure, a journey through an extra-dimension could provide a short-cut through our Universe's spacetime, shaving off those 60 nanoseconds easily. But if those extra dimensions were accessible at these relatively low energies that the OPERA neutrinos have, we would have seen see lots of other telltale phenomena at both the LHC and at Fermilab. The fact that what we've seen at both places agrees so strongly with the simple Standard Model and nothing more -- no SUSY, no Extra Dimensions, etc. -- tell us that string theory is probably not playing a role in this experiment.
Image credit: LNGS.
3.) When the paper was first announced, information about this neutrino experiment was everywhere, and now I have to search to find up to date information about the status of these experiments and the science community's reaction to it. Why isn't the media continuing to cover this story?
The science community is -- rightfully -- skeptical of these results. If they hold up to repeat, follow-up experiments at other locations, such as MINOS in Minnesota and K2K in Japan, this will be very big news. But we've learned all we can learn from OPERA. They see neutrinos arriving 60 nanoseconds early in their detectors, they've been unable to find an error, and other experiments find that OPERA's results, when combined with other predictions of the Standard Model, do not correctly predict what they see. So either OPERA's results are wrong or our current theories about how particles and fields work are wrong when applied to OPERA's neutrinos. If we want to learn more, we need to do something new to find it out, and that's why the media coverage has dropped off.
Image credit: Origin unknown; retrieved from Paranormal Spy.
4.) When the news first broke on the possibility of faster than light neutrinos there was also a lot of talk about the associated possibility of time travel. What do faster than light neutrinos have to do with the theories around time travel?
It's a simple matter of economics: it's cheaper to build the OPERA experiment than it is to perform the annual maintenance on a DeLorean DMC-12.
No, no, I'm kidding. Special relativity tells you that the laws of physics should be the same in all inertial (non-accelerating) reference frames. So if I send a signal from point A to point B, then all observers, no matter where they are, in what direction or how quickly they're moving, will see that the signal was sent from point A before they see the signal arrive at point B. And this is true, so long as the signal moves at or below the speed of light.
But if that signal moves faster than light -- i.e., if the neutrinos arrived at the OPERA detector before a photon moving at the speed-of-light-in-vacuum would have -- then some observers would see that signal arrive at point B before they see you send it from point A. Hence the joke,
We don't allow faster-than-light neutrinos in here, said the bartender. A neutrino walks into a bar.
Image credit: H. Nunokawa, 2000, who took it from Smirnov, 1999.
5.) What was the result of the experiment's initial purpose, to test if one kind of neutrino could turn into another kind, and what are the implications of that result? Could these two different results be related in any way?
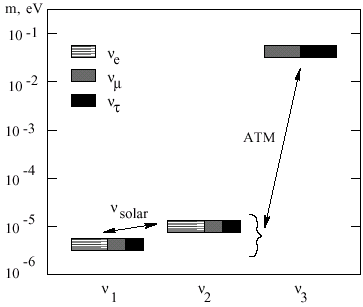
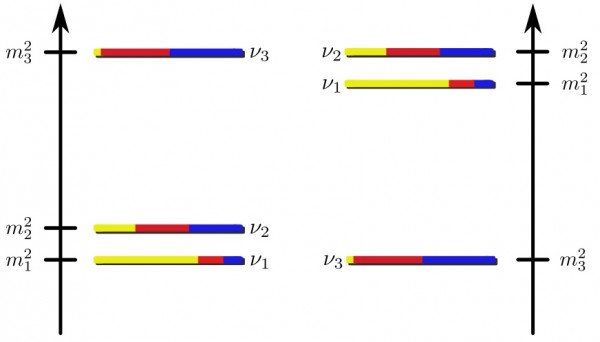
There are three types of neutrinos -- electron, muon, and tau -- but they all have, and this is very important, the same quantum numbers. Same charge, same lepton number, same baryon number, same spin, same isospin, and almost the same mass. (A little more background on neutrinos here and here.) The way it works in quantum mechanics is that if you have the same quantum numbers, you mix together. So there might be three distinct masses for the things that make neutrinos, and we'll give them some clever names, like m1, m2 and m3. (Technically, we call these mass eigenstates.)
What we see as an electron neutrino might be 70% m1, 20% m2 and 10% m3, while the muon and tau neutrinos would have different percentages. Mixing is something that's well known to happen for the weak interactions in both quarks and neutrinos, but it's been much more difficult to measure for neutrinos than it is for quarks. So that was the original goal of this experiment: the measurement of neutrino oscillations was designed to measure exactly how neutrinos (and anti-neutrinos) do their mixing with one another.
Image credit: kismalac on wikipedia.
6.) How can neutrinos oscillate when they have different masses?
So this is actually really interesting: the way that they oscillate between electron, muon, and tau types allows us to determine what the mass differences are between the different types! (Technically, the mass-squared differences.) By measuring the way the different oscillations take place, we can also tell whether there are only three fundamental types of neutrino (there must be at least three) or more than three: a fourth type would mean there must be sterile neutrinos, or a neutrino beyond what the Standard Model predicts! In other words, we were looking for new physics in this arena when we (may have) found it in another.
And finally...
Image credit: Kamioka Observatory, ICRR, University of Tokyo.
7.) Where do the neutrinos that miss the detector end up?
First off, this is almost all of the neutrinos. A little math: we generated about 1020 neutrinos in the OPERA experiment, and about 16,000 of them were caught by the detector, which is 20 meters long on its longest side. So if we wanted to catch half of these neutrinos, we would need about 5 x 1019 of them to interact. Assuming we just stacked identical copies of OPERA, one-after-another, until half of the neutrinos interacted, know how many we'd need?
3,000,000,000,000,000 of them! Or, for those of you who'd rather have one giant detector, you'd need a version of OPERA that was right around seven light years long. Sooooo.... most of them don't miss the detector so much as they simply pass right through it, and they continue to pass through the mountain, through the atmosphere, and go off into space, where they pretty much continue to pass through every star, planet, gas cloud and galaxy they encounter.
Image credit: Steve Crouch, retrieved from cs.astronomy.com.
For what it's worth, a single neutrino would have to pass through about forty million Suns before it had a 50% chance of having one interaction with any of the particles inside.
Don't wait up.
- Log in to post comments
In practice, how does one do this? In particular, how do you determine the plane that contains the arc and its center of curvature?
The popular media seems interested only in sensationalizing novel results, i.e. those that are most unlikely to prevail under additional scrutiny. This will be ignored until sometime in the future when the result is (likely) refuted, after which we will get the inevitable annoying "Scientists Were Wrong!" headline.
Hey Ethan, how 'bout a 101 on the weak force (or is it "electro-weak interaction" now?). I still have trouble with proton decay and radioactivity and fission and fusion and heavy W and Z particles. I've heard that neutrinos get involved with all that -- but very weakly! What more should we non-physics majors know about this stuff? Thanx.
Dr Siegel, greetings from Mexico! Ensenada, Baja California to be exact, city of the highest concentration of scientists in Latin America, thanks to the CICESE institute as well as the UNAM Astronomy Department.
I'm a longtime lurker in your blog, and am in the process of listening to your excellent interview linked here.
Today I feel obliged to comment because I'm confused:
Since the eighties, I remember reading about the years-long Great Neutrino Hunt in water tanks down mine shafts. Then around 1992 if memory serves, I remember reading about how FINALLY there had been a flash down there, and the hunt was finally a success - neutrinos had been proven to exist beyond the theoretical.
Now I'm hearing about the detection of a torrent of neutrinos from the 1987 LMC supernova, when I was under the impression that neutrinos had yet to be detected back then.
Could you please help clear my confusion here?
Regretfully, I cannot ask a good friend who was actually down one of those mine shafts in Alaska back in the day, since she moved back to her hometown of Madrid a couple of years ago.
Nibi @1 asks: In practice, how does one do this? In particular, how do you determine the plane that contains the arc and its center of curvature?
I don't know the precise technique since it's not my field, but the principles are based on freshman level E&M. Presumably you need a second camera, which would allow you to reconstruct the trajectories in 3-D space. (Alternatively, you aim your one camera in the same direction as the magnetic field, but then you can't derive that component of the velocity.) You know the magnetic field (both magnitude and direction). The component of particle velocity parallel to the magnetic field (the z-component) is not affected, so the particle will tend to spiral around the magnetic field. The relevant plane will of course be perpendicular to the magnetic field (the x-y plane), and you can fit the x and y components of the trajectory to a circle. The radius of curvature (in the nonrelativistic limit; it would take me some algebra to derive what happens at relativistic speeds) depends on the particle's charge (you determine the sign by whether it curves left or right; the magnitude is known), mass, and velocity perpendicular to the magnetic field. You also know that momentum must be conserved in the collision (that doesn't constrain the component along the collision direction, but the other two components must sum to zero if we can neglect the proton's initial speed, as is usually the case), and if the neutrino comes from any direction other than +z or -z that gives you a constraint on the z velocity of the products. For a proton, we know the mass a priori. There are probably some other constraints I am missing here.
Juan,
Neutrinos were detected as far back as 1956 by Reines and Cowan. The detectors you are thinking about are probably Kamiokande or IMB - water tanks down mines - which were searching for, amongst other things, signals of the interaction of neutrinos from the sun. The detection of these was "proof" that the Standard Solar Model was not complete rubbish (although that led to the Solar Neutrino Problem and a longer story). We've had big tanks of water down mines since the early 80's (mostly to look for signatures of proton decay) so there were a few kicking around when SuperNova 1987A went off.
I hadn't thought of the neutrino beam flying off into space through the planet. Would that be detectable by some aliens light years away if they were in the right place?
Good summary, but you did not really answer this question:
"What was the result of the experiment's initial purpose, to test if one kind of neutrino could turn into another kind, and what are the implications of that result? Could these two different results be related in any way?"
Maybe there are no other results published yet?
You also didn't really answer this one:
"How can neutrinos oscillate when they have different masses?"
I believe the question aims at issues of conservation of mass/energy, and it would be interesting to know how we believe this plays out.
b.
>Maybe there are no other results published yet?
They haven't got enough events to check yet. Yes, flavour oscillations can be affected by the speed of light issue - but in any number of weird theoretical ways which require data from new experiments. Besides, other oscillation experiments have been done which fits happily with standard model theory so if the effect is there it is small.
> How can neutrinos oscillate when they have different masses?
Because what oscillates isn't the mass, its the flavour. Think of the neutrino as being created with a perfectly respectable mass, conserving energy-momentum, and travelling to the detector with that mass and energy. However, the flavour of the neutrino (e-ness or mu-ness or tau-ness) changes as it travels. The neutrino mass is so small that we can never measure it (which quantum mechanically is why the oscillation happens) so all we see is something with a predictably changing flavour.
How can anyone be sure that the distance measurement in the OPERA experiment does not have an error? After all, both source and detector are several km below the surface. Any distance measurement must be indirect, and could have any number of accumulated errors.
Is anyone planning to re-do the neutrino travel time experiment with the Ice Cube detector near the South Pole? This detector will give the longest possible distances available on Earth, and is also the biggest detector in the world.
I've read that the precision involved in aiming the LHC beams is so great that even the tidal effects of the moon upon the bedrock in which the track is embedded must be accounted for. I wonder if said tidal effects would be enough to somehow shorten the neutrino path?
Assuming the effect is real (for now):
Do we have any evidence that neutrinos experience gravity? (My guess: no.) Is it possible that the time-dilation effect of a gravitational field doesn't apply to neutrinos? (Observation: yikes, that would be interesting to probe black holes.) If this were the case, would it be consistent with SN1987 neutrino detection? Would it be enough to explain the 60 ns of OPERA?
Questions for experimental work: How short a path can we measure this arrival discrepancy over (assuming it's the size OPERA indicated)? Is it possible to sense it much closer to CERN? Would an experiment at 12km be able to measure a 1 ns discrepancy?
I am not a physicist, so please don't laugh at me.
1. c is the "speed of light in a vacuuum."
2. Their is no vacuum because of virtual particles, gravitational and electromagnetic fields,properties of other dimensions.
3 therefore our measure of c is actuall < the actual theoretical c.
4. nutrinos interact much more weekly than light and therefore are not slowed by the enviroment as mush a is light.
5. Therefore it is possible that nutrinos measure to be faster than c its speed is actually slower than the theoretical speed of light which would be > c.
Another thing about GPS (special relativity is not a problem since it is predictable) is that the signal propagation time through the atmosphere changes and is affected chiefly by water vapor. This is one of the (many) reasons why more satellites = better estimate of time and position.
I'm yet to be convinced of (1) the estimate of the distance between source and detector and (2) that the time is sufficiently synchronized between source and detector. One way to unambiguously determine if there is a timing offset of some sort is to build a second OPERA detector at least twice the distance away from the source. It's an awfully expensive way to reject the FTL claims but the results will be indisputable.
Eric Lund @4:
Thanks, that helped clarify things for me - it's been a long time since freshman E&M :). I tried to formalize what you were saying using qv X B = ma and ended up with a simple system of equations that gave
vz = constant
and (vx)^2 + (vy)^2 = constant
for B=(0,0,constant).
which is a spiral that projects to a circle in the x-y plane as you described (the relativistic factor for mass canceled out).
Fantastic discussion
My father Robert(NMI) Jackson and I were
discussing similar conjectures in 1991
that if we ever discover a faster than light
sub particle we should attempt to
send simple code with this sub particle
the longest distance possible because
it might be detectable in another universe
@11 Joffan
"Do we have any evidence that neutrinos experience gravity? (My guess: no.)"
If they didn't it would be a phenomenon on it's own. I mean, they have mass, hence there is gravity. Gravity is curving of spacetime caused by mass. So for anything having mass not to be affected by gravity would be a paradox indeed.
@11 Joffan & @16 Sinisa Lazarek:
For anything having mass to not be affected by gravity would mean the Equivalence Principle, one of the core principles of General Relativity, would not apply to neutrinos.
Heck, even 'massless' particles such as photons are affected by gravity. (See 'gravitational lensing'.) Of course, this is because 'massless' only means no rest mass; photons still have energy, and energy is equivalent to mass, and that mass/energy is affected by gravity.
Thanks for the responses - yes indeed, that would mean breaking the Equivalence Principle. Gravitational mass and inertial/energetic mass separated at last, for one category of almost non-interacting particles. Perhaps - more devil's advocacy - that's why they don't interact much.
Hi Ethan,
I have a remark that falls in the category 'attention to detail'. It's about relativistic time on satellites.
The background to my point:
Historically, the first time relativistic effects were elicited in experimental setups was the case of relativistic velocities in a cyclotron. To explain the effect in that type of setup it suffices to present special relativity.
It is a historical artefact that we tend to associate velocity time dilation with special relativity only.
Now to my point:
As we all know, general relativity subsumes special relativity. General relativity accounts for all relativistic effects.
In the case of the GPS satelites we have that there is in fact a single relativistic effect, described by general relativity.
But it is ever so tempting to dissect into two effects; one a velocity time dilation effect, and the other a gravitational time dilation effect. However, this dissection is an artefact. It's not inherent in the physics.
Quite a few popularizers present such a dissected picture, suggesting that special relativity and general relativity are acting independently. Which, given that GR subsumes SR, amounts to self-contradiction.
Audience members tend to phrase questions in terms of an involvement of special relativity all by itself ("Aren't the GPS satellites subject to special relativity?")
You don't have to go along with that mistaken perception. While you can still mention velocity effects and gravitational effects, you can steer in the right direction by simply stating that both are accounted for by relativistic physics.
The clearest exposition of this point, I think, is by Kevin Brown, back in 1997, in a Usenet post
http://groups.google.com/group/sci.physics/msg/53ae16d3ff8a8217?hl=en&d…
Another excellent post!
Steven Boyd,
Thank you for your answer, which clears up a few things, but a nagging question remains:
It took years of vigilance to detect the first solar neutrino from 8 light minutes away. Several years before, a wave of neutrinos from a distance 160,000 light years seems to have been detected without breaking a sweat.
From my layman perspective, this doesn't make sense, because the solar neutrino detection initiatives, as I understood them, gave the impression that no instrument above ground could detect these almost massless particles, amid the noisy surface "leptonic bombardment", to coin a term.
Complicating clarity further, there's no comparison between a short neutrino burst from the LMC, and a constant barrage from our sun.
But the LMC burst arrival was detected in real time,
yet the constant sun stream took years to confirm.
- - - - -
As for the Solar Neutrino Problem, that would be about the proportion of tau to muon, the possibility that neutrinos flip from one state to another, wouldn't it? And that would be one of the primary goals of research in Gran Sasso, but that's taken a turn for the supremely weirder.
I know why neutrinos are faster than light and this is my theory...
http://sagargorijala.blogspot.com/
I created the theory after reading Prof.Stephen Hawking's book... A Brief History Of Time.
I created the first postulate and the postulate that explains why neutrinos are faster way back in January 2000.