“Exploring this set I certainly never had the feeling of invention. I never had the feeling that my imagination was rich enough to invent all those extraordinary things on discovering them. They were there, even though nobody had seen them before. It’s marvelous, a very simple formula explains all these very complicated things. So the goal of science is starting with a mess, and explaining it with a simple formula, a kind of dream of science.” -Benoit Mandelbrot
Sure, there are things we can say or describe, whether mathematically, physically or in words. But sometimes, you need a lot more than that to really understand what's happening. Have a listen to Yo La Tengo’s remarkable song,
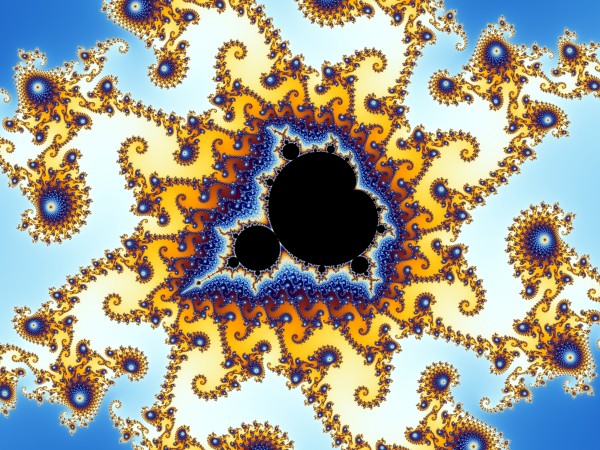
while you consider a dive into the most important set in all of fractal geometry: the Mandelbrot set.
Image credit: Wikimedia Commons user Wolfgangbeyer.
Sure, I can (and will) tell you all about the mathematics of it, including self-similarity and what defines this complex picture, as well as how it compares to the observable Universe.
But is that really going to help you understand it as much as watching an animated simulation, diving in hundreds of orders of magnitudes?
Image credit: YouTube channel Fractal universe, via https://www.youtube.com/watch?v=zXTpASSd9xE.
Go watch the compilation I've put together, and enjoy these stunning visualizations this weekend!
- Log in to post comments
More like this
I was hoping that the article would say which specific point the video is zooming in on, but it does not. I have a guess that the point selected is a pair of rational numbers with at most 120 significant digits each.
The point it is zooming into is a point on the complex number plane, and the real and imaginary components will be real numbers, rather than rational. As to 120 digits, around 20 years ago the program Fractint was able to handle numbers large enough to handle a zoom of 10^1600. Math is much, much bigger than physics.