When it comes to Einstein, the first thought that comes to people's mind is usually relativity -- either special or general -- or perhaps his famous E = mc^2. (Except for Anupam Garg; my old classical mechanics professor used to joke that Einstein's greatest contribution to physics was his shortened summation notation.) But Einstein's legacy goes much deeper than any of that.
You see, one of the greatest things that Einstein pioneered was the Gedankenexperiment, or the idea of performing an experiment in your mind. These weren't fanciful, impossible-in-principle experiments, but rather ideas that could someday be tested, and that we could set up in our imaginations before the technology ever got there. This idea has led to some of the greatest paradoxes in scientific history, many of which have pointed the way forward to the next frontier in physics.
Sabine Hossenfelder has a fantastic post on the subject; check it out here!

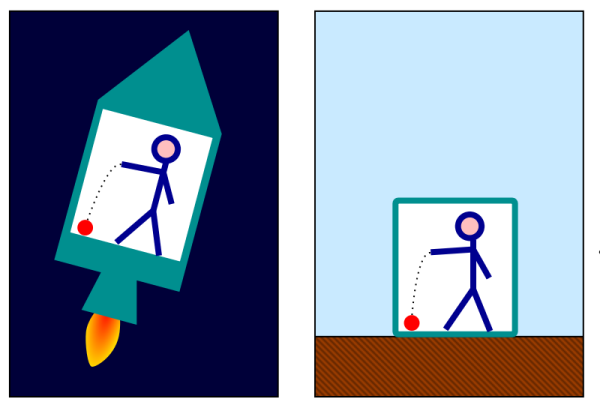

There's some things in this piece that a relativist might not like. For example, the equivalence principle only applies to an infinitesimal region. Which means it doesn't apply to the room you're in. Because it's a guiding principle, not something that says a genuine (Einstein said "special") gravitational field is exactly the same as being in an accelerating rocket. Also note this:
1.What is relevant is only that what is measurable.
That raises an eyebrown, because sometimes when you measure a change, it's because the thing changed. But sometimes it's because you changed. And sometimes when you don't measure a change, the thing changed, and so did you. Now look at this:
2.You must not fool yourself.
If you go to a lower location, you measure a photon as blueshifted. But it hasn't gained any energy. Instead you lost it. You know the photon hasn't gained any energy because when you send a 511keV photon into a black hole, the black hole mass increases by 511keV/c². Not a gazillion tons. Also see this:
He had Michelson-Morley’s experiments that disproved the aether
Einstein reintroduced an aether for general relativity. See his Leyden Address and look on arXiv. Sorry to be pernickety, but I do think it's important to get this stuff right.
@John Duffield #1: You write, "[T]he equivalence principle only applies to an infinitesimal region. Which means it doesn’t apply to the room you’re in." I think that's an over-generalization in the context of actual measurements.
Specifically, the equivalence principle applies to the Minkowski frame tangent to a specific point in curved space-time. As with *ANY* use of a tangent as an approximation to a curve, the most important quantity is not delta and epsilon, but rather the sagitta. How far away from the tangent point do you have to get before you can actually _measure_ the difference?
In the case of Einstein's elevator, the answer to that question is very simple. Most macroscopic gravitational fields are central, so the "maximum width" of the elevator is determined by when you can measure that a body falling near the wall isn't really moving perpendicular to the floor, but has a small inward shift (because of the radial force).
I will leave it to your mathematical skill to determine how big a room on Earth would have to be before you could measure the effect. Here's a hint -- the answer is bigger than a house.
That sagitta relates to tidal force, which relates to Riemann curvature, which is the "defining feature" of a genuine/special gravitational field. Einstein was clear that you cannot transform it away, see section 20 of Relativity: the Special and General Theory:
"We might also think that, regardless of the kind of gravitational field which may be present, we could always choose another reference-body such that no gravitational field exists with reference to it. This is by no means true for all gravitational fields, but only for those of quite special form. It is, for instance, impossible to choose a body of reference such that, as judged from it, the gravitational field of the earth (in its entirety) vanishes".
This is why the room you're in is not equivalent to the room in the rocket. See the plot of gravitational potential on Wiki. It's like the Riemann curvature depiction of curved spacetime. Your reading of the equivalence principle does away with the curvature, so the plot can never get off the flat and level. So you've thrown the baby out with the bathwater, and there's no gravitational field at all. For Einstein's real legacy, see Baez and note this:
"Einstein talked about the speed of light changing in his new theory. In his 1920 book "Relativity: the special and general theory" he wrote: "... according to the general theory of relativity, the law of the constancy of the velocity of light in vacuo, which constitutes one of the two fundamental assumptions in the special theory of relativity [...] cannot claim any unlimited validity. A curvature of rays of light can only take place when the velocity [Einstein means speed here] of propagation of light varies with position." This difference in speeds is precisely that referred to above by ceiling and floor observers".
Let's see, whom to believe, Ethan and Michael or John.
Put that way it's an easy decision - believe the physicists.
@John Duffield #3: John, you seem to have misunderstood my response. I was not in any way claiming that the curvature of space-time can be "transformed away." I made a statement about _measurability_, which you have conveniently ignored.
Once again, I want you to perform a very simple calculation. Suppose you drop identical weights inside a room (an office, a gymnasium, a football stadium, whatever you choose). Hold the weights exactly one meter above the floor and drop them, making as careful note as possible of where you started them and where they landed.
Now for the calculation. How far apart do the weights have to be, before you can MEASURE the fact that they fell along slightly converging radii for that one meter distance?
If you cannot MEASURE the convergence, then your room, for the duration of the experiment, is equivalent to a uniformly accelerated box.
If you are unable to do the calculation, please just say so.
@Michael Kelsey #5,
I'm certainly unable to do the calculation for that experiment, so I thought of another.
If you place two vertical spans of two meters length two meters apart at or near Earth surface, the distance at the top is about 630nm more than it is at the bottom - that's one full wavelength of orange light.
The question then becomes how close to vertical one can make a span.
I'd understood that one of the earliest useful Gedankenexperiments was Gallileo deducing that all bodies fall equally fast. He imagined the tension arising in string connecting bodies of different mass, and guessed it couldn't be so, otherwise there'd be macroscopic consequences which would be observable.
The story of cannon balls being dropped off the leaning tower is just that.
@CatMat #6: That's exactly the same calculation! Since we define "vertical" as "parallel to a freely hanging plumb line", those two parallel spans are both pointed in their locally radial direction (of course, we're both making the spherical-cow approximation, neglecting all of the Earth's inhomgeneities :-).
So it might be possible in principle to measure the half-micron difference in a typical room, but not without some serious equipment :-) Einstein's gedankenexperiment still works.
Michael: I didn’t conveniently ignore what you said. I said convergence* is irrelevant, and to reiterate: what is relevant is “Riemann curvature”, which is associated with the second derivative of potential and tidal force, or g at the ceiling as opposed to g at the floor. And regardless of whether you can measure it, you room isn’t equivalent to the accelerated box, because without it there is no change in gravitational potential and so no gravitational field. Look at a depiction of gravitational potential. The first derivative, the gradient, the slope is like this _ in the centre, and like this / at the surface of the Earth. The steeper the gradient the stronger the local “force” of gravity. But without that curvature the gradient can’t change from this _ to this /. No curvature, no gravity. Have look at what Synge said about the equivalence principle being the “midwife” who should be laid to rest. See for example this.
* no I can't calculate it offhand. I could look it up, but it would serve no useful purpose.
I have to jump in because arguing about the room in rocket being same as room on earth was never what the thought experiment was about. And curvatures and geometry only came later on as a mathematical solution. The point is that constant acceleration = being in a gravitational field. Not that intensity is the same, or direction or anything of those later things, but the realization that objects are "free falling" in a earth's gravitational well and that it's the same kind of thing (not similar... SAME) as being constantly accelerated.
There is one error in the article itself IMO, and it doesn't deal with relativity but QM. " In a seminal paper from 1935, the three physicists showed that the standard Copenhagen interpretation of quantum mechanics has a peculiar consequence: it allows for the existence of “entangled” particles."
Entanglement was known before EPR wrote their paper. And it's not one interpretation that allows, it's all of them. The different interpretations explain it differently, and EPR paper is in now way supportive of Copenhagen interpretation. If one is to be honest, the whole point of EPR paper was to show that Copenhagen interpretation is incomplete. Einstein himself was more of a hidden measurments/hidden variables camp, believing in fully deterministic universe. Even today the debate is open.
Was Einstein involved with entanglement? Yes. Is it an effect of one type of interpretation? No! Was Einstein somehow supportive of QM and Copenhagen interpretation in general? Hell, no!
John, one word for you may clear the problem you've got:
Uncertainty.
If you can't measure a difference, then there's "no difference".
The basis of the uncertainty principle and the source of its effects.
Sinisa: being subject to constant acceleration isn't the same as being in a gravitational field. In the former situation you are constantly changing your speed through homogeneous space aka flat spacetime, in the latter you are motionless in inhomogeneous space aka curved spacetime. You might not be able to tell the difference, but that doesn't make them the same.
@ John
"being subject to constant acceleration isn’t the same as being in a gravitational field. "
.. and if you devise an experiment to differentiate the two, you will get a Nobel. Best of luck
@Sinisa Lazarek #13: John and I have already pointed the correct _in principle_ way to distinguish uniform acceleration from a physical (i.e., central) gravitational field. Namely, that the accelerating elevator is missing both the radial gradient (the tidal component of gravity) which John mentioned, and the slight convergence of verticals due to the radial force itself, which I described.
@John Duffield #12: Your last sentence is itself the key. The point of Einstein's thought experiment was _NOT_ that the two cases are the same -- they are trivially not the same for exactly the reason you mention. Einstein's point was that in the thought experiment, the observer IS NOT ABLE TO TELL THE DIFFERENCE. And as I have described, that is a quantitatively verifiable statement, specifically for the case of weak gravitational fields like the Earth's. Einstein's key insight was to realize that the inability TO TELL THE DIFFERENCE is actually a very subtle and very important feature of nature: it means that the constant of proportionality for inertia is the same as the "quantity of matter" which appears in the gravitational equations. Those two parameters didn't have to be the same, but the equivalence principle requires that they are.
@ Michael and John
While both of you have much more mathematical background, and while Michael, of course, has much much more physics background... when it comes to equivalence principle, I don't feel that the impact of the idea is suitably appreciated.
John still feels he understands Einstein better that Einstein himself. So i will quote Einstein (from wiki here).. concering two systems (one in grav. field, and other accelerated reference frame)
" But this view of ours will not have any deeper significance unless the systems K and K' are equivalent with respect to all physical processes, that is, unless the laws of nature with respect to K are in entire agreement with those with respect to K'. By assuming this to be so, we arrive at a principle which, if it is really true, has great heuristic importance. For by theoretical consideration of processes which take place relatively to a system of reference with uniform acceleration, we obtain information as to the career of processes in a homogeneous gravitational field."
And this is why i feel "is not able to tell the difference" is not giving enough creedence to this. They are EQUIVALENT in respect TO ALL PHYSICAL PROCESS!
This is the deep insight. Yes, rotating massive bodies impart other things from relaitivity.. frame dragging, tidal forces etc... but those come later on. Just like curvature comes later on. If inertial mass wasn't the same as gravitational mass.. things would be different in physics.
Michael: Einstein certainly spoke of his "happiest moment" when considering the man falling off the ladder. But IMHO there was some evolution going on in his thinking.
Sinisa: I don't feel I understand Einstein better that Einstein himself. I just have the benefit of his later work which he didn't have when he wrote his earlier work. For example if you look at his 1920 Leyden Address you can see him referring to a gravitational field as inhomogeneous space, which doesn't sit well with a "homogeneous gravitational field". That's what Synge was on about. See the quote on page 20 of this essay, where there's also a quote by Ray:
"It is very important to notice that in a freely falling frame we have not transformed away the gravitational field since the Riemann tensor (gravitation Riemann tensor) will not vanish and we will still measure relative acceleration ….. The first thing to note about the 1911 version of the principle of equivalence is that what in 1911 is called a uniform gravitational field ends up in general relativity not to be a gravitational field at all – The Riemann tensor is here identically zero. Real gravitational fields are not uniform since they must fall off as once recedes from gravitating matter."
@ John
"I just have the benefit of his later work which he didn’t have when he wrote his earlier work."
then you can rephrase it circa 2014, and it's still be the same
"The local effects of motion in a curved space (gravitation) are indistinguishable from those of an accelerated observer in flat space, without exception."
as for slope in the gravitation field of massive bodies, that is not what is argued. At any point in the slope, or at any distance of the center of mass, the field has a certain value. accelerate a refrance frame in flat space and you must get the same result.
... or alternatively.. if there is a difference in measurment, there is an additional force in the universe and can't be explained by gravity alone.
On Gallileo and thought experiments, see this:
http://www.philosophical-investigations.org/Galileo%27s_Thought_Experim…
Sinisa: see Baez where you can read this: "Similarly, in general relativity gravity is not really a `force', but just a manifestation of the curvature of spacetime. Note: not the curvature of space, but of spacetime. The distinction is crucial." I've told you about this before, see this. And yet you continue to say things like “the local effects of motion in a curved space (gravitation) are indistinguishable from those of an accelerated observer in flat space, without exception.” A gravitational field is where space is inhomogeneous, not curved. We model motion through it over time as curved spacetime. Not curved space. Standing motionless on the Earth is somewhat like accelerating through free space, but it isn't the same. Ignorance and misunderstanding such as yours is why I make the comments I do. Try to take some of them on board.
John, you can do a 3+1 decomposition in any curved spacetime and find that space, in fact, is curved as well. This is true any way you slice it.
Baez's point is that you must consider the time dimension to get an acceleration and therefore what manifests as a force. But you can do this by considering it as either a 4-d spacetime or a 3+1-d space+time concept.
Perhaps that's exactly the point you were trying to educate Sinisa on, but your comments didn't come across that way.
John you owe everyone an irony meter.
This essay is rather superficial, because Einstein wasn't indeed first, who introduced the thought experiment into science. The ancient Greek "deiknymi" was the most ancient pattern of mathematical proof, and existed well before Euclidean mathematics. Perhaps the key thought experiment in the history was Galileo's demonstration that falling objects must fall at the same rate regardless of their masses. This experiment is described by Galileo in Discorsi e dimostrazioni matematiche (1638) in form of discussion.
/* "He had Michelson-Morley’s experiments that disproved the aether"*/
This is also solely wrong - this interpretation is, it just puts the luminiferous aether concept on its head and it contributes to the widespread misunderstanding in this way.
The reference frame of luminiferous aether cannot be determined until such an aether really forms the space - i.e. not just pervades it as a sparse gas. If something forms the space, it cannot be detected with motion of this space, with its transverse waves the less. The transverse waves have no reference frame in any medium.
The problem of gedanken experiments is, they cannot lead to correct solution, when they're based on false premises, or when such a premises have holes (like the famous Feynman "beads of stick" gedanken experiment, and many others).
For example, in real observable Universe it's not quite true, that "..to an observer inside the elevator, one cannot tell, by any possible measurement, whether the elevator is at rest in a gravitational field or is being pulled up with constant acceleration"
It's true only for diehard fan of general relativity (which Mr. Einstein undoubtedly was) - but it has a very simple limitation, if you can think about it in open-minded way - or if you can measure whether or not the field is exactly parallel. This doesn't mean that GR is wrong, though, only that the equivalence principle applies in the limit of a vanishingly small elevator.
So that the gedanken experiment isn't any miraculous solution for how to avoid the logical errors in your deductions. It's just a mental tool like any other - and you should handle this tool with caution.
The actual rôle of thought experiments in the history of physics seems quite different to what your and Sabine Hossenfelder's (otherwise, as relates to non-historical questions of physics, very instructive) blog suggests.
Here is a brief outline of the history of the concept "gedankenexperiment":
http://philsci-archive.pitt.edu/4700/