Comments of the Week #47: From parallel yous to fundamental constants

“And there is the headlight, shining far down the track, glinting off the steel rails that, like all parallel lines, will meet in infinity, which is after all where this train is going.” -Bruce Catton

At the end of each week here at Starts With A Bang, it's important to take a look back at all we've gone through, and give some time and energy to all your thoughts on it. This week, there have been a lot of them, as we've covered the following:

• The multiverse and you (for Ask Ethan),
• Revenge, it's what's for lunch (for our Weekend Diversion),
• The galactic plane (for Mostly Mute Monday),
• Dark matter can give you cancer -- and that may be a good thing (by Sabine Hossenfelder),
• Cosmology in the 21st Century (a live-blog experience of a Perimeter Institute talk),
• The strangest moon in the Solar System, and
• The fundamental constants behind our Universe (for Throwback Thursday).

That's a huge amount of material, and you've responded with over 100 comments on these articles to choose from. I've had to leave out some really good ones, so don't be sad if your favorites didn't make the cut. That said, here are your Comments of the Week!

Image credit: Ozytive, via http://www.ozytive.com/wp-content/uploads/2013/09/Parallel-Universe.jpg.

From UncleMonty on signatures of other Universes in our own: "“As time goes on, because of the dynamics of expansion, no two regions where inflation ends will ever interact or collide”. Is this not controversial? I remember reading in the past 2 or 3 years that some astronomers were searching for the signature of cosmic bubble collisions, where our universe *had* in fact collided with another nucleating bubble."

Kind of. It was Roger Penrose who said it, and it was known to be wrong when he said it, despite many (such as Matthew Kleban) also making claims to the contrary.

Image credit: Roger Penrose and Vahe Gurzadyan.

While bubble wall collisions would, in fact, leave that concentric-ring signature which have never been found, they should not be produced in inflation. Think about a pot of boiling water. You get little bubbles of air that form on the bottom, and those bubbles grow over time. As they grow and rise, the bubbles nucleate, interact and grow, merging together to form larger bubbles.

Image credit: cosmology.com.

But in an inflationary Universe, the portions that are inflating expand exponentially, while the portions where inflation ends -- the bubbles -- can only expand outward at ever-diminishing speeds, while the matter and radiation within them are limited by the speed of light.

If you form two bubbles that are not initially connected, they will never merge together or collide. That's not speculation; that's the physics of the inflationary Universe, and it's well-known. It is, in fact, the biggest problem with Guth's original inflationary model, where even he -- in his original paper -- realized that there was no way to create an isotropic and homogeneous Universe if the bubbles in his model didn't collide, and they couldn't! Thankfully, new inflation came along, and that problem has since been solved. But it isn't by concocting a fix to get the bubbles to collide, but rather by finding a model where bubble collisions were unnecessary.

Image credit: NASA / GSFC, via http://cosmictimes.gsfc.nasa.gov/universemashup/archive/pages/expanding….

From G on the same post: "Question: How recently did “inflation preceded the Big Bang” make it into the canon?"

Honestly? When the COBE observations validated inflation, it became Universally understood that inflation was the thing that set up -- and hence preceded -- the hot Big Bang. So that was 1992 when the first compelling COBE results came in, and those who adhered to inflation since the early 1980s were suddenly validated. This has been well-known and accepted in the cosmology community ever since.

Image credit: Takeo Moroi & Tomo Takahashi, from http://arxiv.org/abs/hep-ph/0110096; annotations by me (in blue).

Now, some people don't mean the "hot Big Bang" when they use the term "the Big Bang," but rather mean "the birth of space and time itself." In that case -- which assumes there is a birth to space and time -- there is nothing that can precede it by definition, even though nothing is big and nothing is banging when that occurs.

On a personal level, I think that physicists who adhere to that latter definition, pardon my French, are still clinging to pre-1980s definitions and assumptions, and need to enter the current generation. They won't, though. As Max Planck famously said, physics advances one funeral at a time!

Sketch of the proposed experiment. An incoming dark matter particle kicks out a gold atom which goes on to break several strands of DNA.

From Denier on detecting dark matter using DNA: "Interesting concept but my first question was how they planned on differentiating DNA damage done by DM versus DNA damage done by neutrinos. Upon reading the paper, the answer may be that they can’t tell the difference but I give them bonus points for honesty."

This is an interesting question, because for any one individual event, you can't tell. The only information you're going to get out -- because you can't detect the dark matter particle directly -- is about the energy and momentum that gets imparted to the gold atoms that wind up running into the DNA. So for one event, you can't look back and say, "that was a dark matter particle," or, "that was a neutrino." You can't know.

Image credit: cross section for atmospheric neutrinos; Takaaki Kajita, New J. Phys. 6 (2004) 194.

But in particle physics, the energy and momentum you get as a result of a collision have everything to do with the initial mass and energy/momentum of the incoming high-energy particle. If you start collecting a large number of events, you can reconstruct what the spectrum of events should look like, and if you have an excess in a certain part, that can be evidence of new physics, or even a new particle.

That is the great hope of what we'll find: we know what the neutrino signature ought to look like, and hence we need to find an excess of events where there shouldn't be any. Different particle properties for the dark matter would leave such a signature, and that's what we're hoping to find!

Image credit: Perimeter Institute.

From Laurie on Cosmology in the 21st Century: "Will there be a VoD (Video on-Demand, for later viewing)? If so, where would we find the link?"

Perimeter Institute was kind enough to invite me to not only do a live-blog and live-host of the event -- which they told be brought them many hundreds of new eyes (they track it) during the event -- but they encouraged me to to host the final, recorded-and-edited event from start-to-finish over at my main site.

Image credit: Screenshot from the live webcast event.

There, you'll find not only the whole 1 hr 13 minute talk plus the Q&A, but also the entire live-blog commentary I wrote, so you can scroll down, pause the video at any time and follow along for additional commentary.

I think you'll like it, and -- perhaps just as importantly -- Perimeter liked it so much that they've promised to do the same thing with me again in the future the next time they have either a space/astrophysics or theoretical physics lecture topic come up!

Image credit: NASA / JPL-Caltech / Space Science Institute / Cassini.

From eric on Iapetus, the Solar System's (according to me) strangest moon: "Here is an article from last year discussing Iapetus’ equatorial ridge. Looks like its dust, not science, that has settled…"

The link eric points to suggests that -- since there are no tectonic plates or cooling effects that could have explained the ridge -- it's conceivable that dust falling on the equator from a non-Iapetus-body could have caused it.

As far as we can tell, it could have, but this isn't compelling evidence that this idea is correct. In fact, there are at least two competing ideas that are just as plausible, if not more plausible.

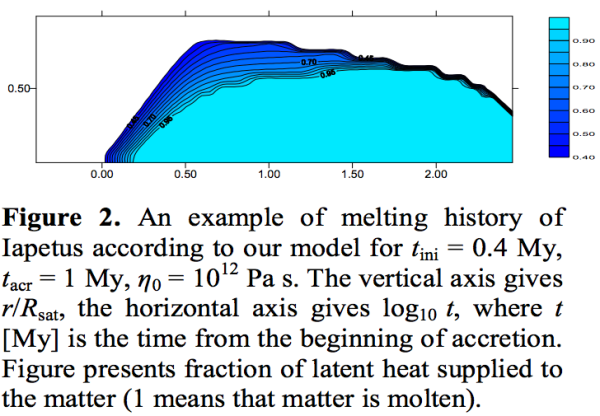Image credit: L. Czechowski, J. Leliwa-Kopystynski, ESPC, 2012, via http://meetingorganizer.copernicus.org/EPSC2012/EPSC2012-834.pdf.

My favorite of these is that Iapetus started out with a faster rate-of-rotation than it presently has, just as Earth started out with a rate of rotation that's approximately three-to-four times its present rate. Iapetus now has a rotational period of 79 days, being tidally locked to Saturn, as all moons appear to be locked to their parent gas giants. But if it initially had a 17-hour rotational period after forming, that could explain the height of the ridge. Radioactive heating from isotopes such as Al-26 could have allowed it to remain hot enough to plastically deform for millions of years, which could have enabled its present, unique shape.

The point, though, is not to decide right now which of these ideas is correct, as we don't have sufficient evidence to do that, but to hold all the plausible ideas in our heads, and keep in mind what evidence could come in to allow us to validate or falsify various models. That's the real way that science progresses, and that's how we get it right in the end!

Image credit: NASA / CXC / M.Weiss.

And finally, a very difficult question from the ever-circumspect Michael Kelsey on the fundamental constants of our Universe: "But if you’re talking about simualtions like Millenium or VIa Lactea, the fundamental constants are put in by hand, so they can’t change. I’m not familiar with a large scale cosmological simulation which has tried to deal with time-varying constants.
It seems to me that we really _don’t_ know how likely our particular set of constants is. Since you’re the astrophysicist, if I’m wrong, could you point me toward a decent review?"

To first give credit to Michael, he's a particle physicist, and could very likely have given a much better answer to the dark matter particles vs. neutrinos question asked earlier than I did.

As far as this question goes, there is an assumption that I made, that so far is backed up by the evidence. But that isn't necessarily true.

Image credit: 2dF galaxy redshift survey.

I have assumed that the fundamental constants of the Universe are exactly that: constants, and that they are the same at all places and at all times in our Universe. Because of the level of isotropy and homogeneity we observe -- how the laws and properties of the Universe are the same in all places and directions -- we have a very strong observational constraint that most of the fundamental constants are (on average) constant to about 1-part-in-1,000,000, over timescales stretching back many billions of years and tens of billions of light-years. (The Webb articles on time-varying alpha are both right at this limit, and are also highly contested.)

Image credit: ESA and the Planck collaboration, modified by me.

We also know, in the very early Universe, that the temperatures got up to somewhere around 10^15 GeV, or about a factor of 100,000,000,000 larger than the maximum energies we'll reach at the LHC. And yet, it appears that the constants all settled into the same values everywhere. This would be like taking a rock 100,000 miles into space, high above Earth, dropping it, and seeing it fall into a valley. Then taking an identical rock and performing this experiment quintillions of times, and seeing it land in the same spot in the same valley every time.

I can't be certain that the constants aren't different in some places, or that they haven't changed over time, but based on what we do see, it's my suspicion that there are some underlying dynamics that caused them to have the observed-to-be-uniform values that they have in the Universe. And that's the best answer I have for you.

Thanks for a great week and a great selection of comments, and I hope I did them justice for you. See you back here next week!