“The radiation left over from the Big Bang is the same as that in your microwave oven but very much less powerful. It would heat your pizza only to -271.3°C, not much good for defrosting the pizza, let alone cooking it.” –Stephen Hawking
Imagine you traveled out into empty space. Away from any and all planets, stars, galaxies, and matter in general: normal or dark. Would you simply find yourself immersed in an empty, energy-free abyss? Not so! You'd still be bathed in radiation: not just from distant starlight, but from the afterglow of the Big Bang itself.
Image credit: ESA and the Planck Collaboration.
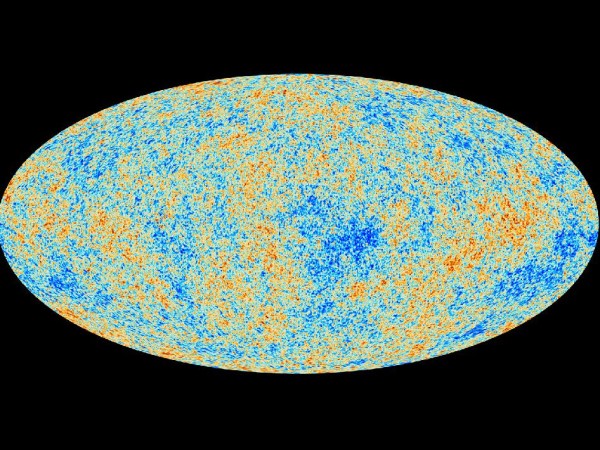
That's the cosmic microwave background. What you might not realize is that all by itself, it tells us what the Universe is made out of today. Not only that, but there are anomalies in the radiation itself. Does that mean new physics is present? Or are the anomalies not so anomalous after all?
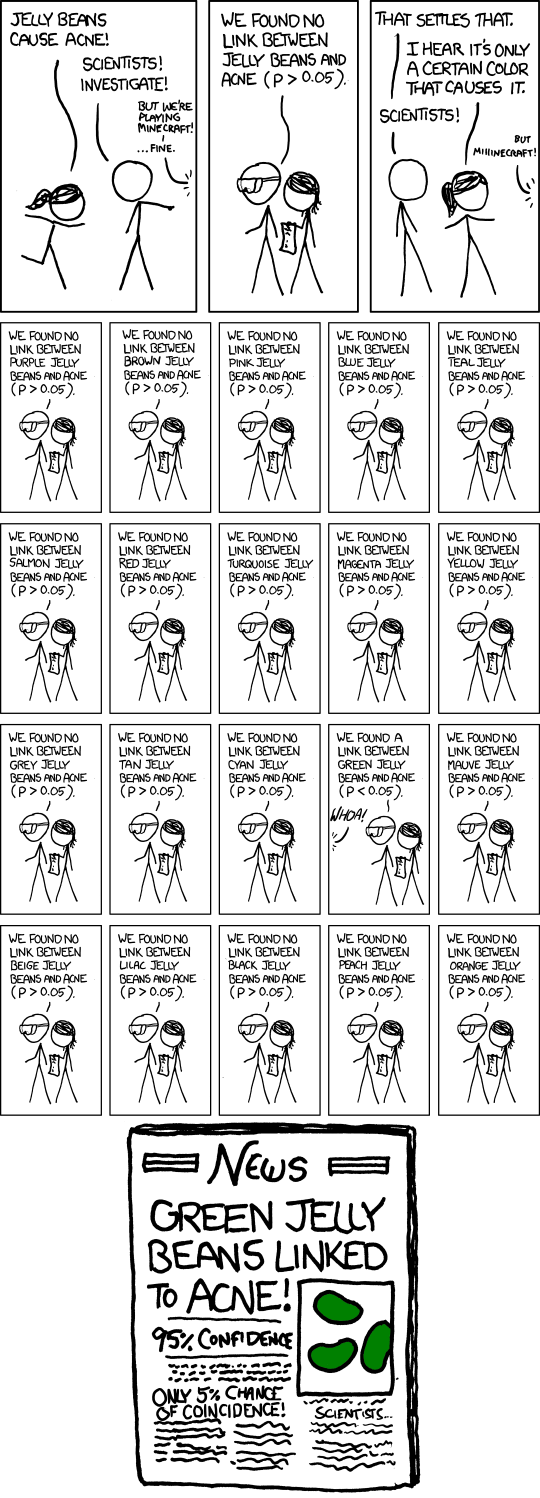Image credit: Randall Munroe / xkcd, via https://xkcd.com/882/.
Come find out, and get the science of the cosmic microwave background!
- Log in to post comments
I wonder how long it will be before this topic gets bombed ......
PJ, you know what you just did, right?
For someone who goes "Hey, if you don't mention it, it will go away", you really don't listen to yourself, do you?
If that isn't enough for you, or you think it just fine, then ask yourself this: what did your post have to say on this topic?
Nothing.
Right?
So. Why?
PJ, try and accentuate the positives.
These threads/topics from past could possibly hold records for not succumbing to Godwin's law.
That's a positive no?
Getting moderated now, I bet WOW isn't :-)
How do these mapping results differ from what we should detect from such devices if we may assume what they detect is derived at microwave bandwidth from the net vacuum energy of (approaching) infinite electromagnetic sources degrading across (approaching) infinite space? This does not admit itself as a relic of any single event but rather as the expected limit of such an investigation. IMHO
"Getting moderated now"
Apparently not.
"I bet WOW isn’t"
Paranoid delusions and conspiracy ideation.
Lloyd, the answer is that the variations of the background are commensurate with the vaccuum fluctuations of inevitable inflation with the paradigmatic laws in-place that result in this reality of stability. Therefore there is no discrepancy to be expected in the patterns of inherent energy of space in the collapsed manifold of three-space.
Yeah had to do a little IP dance to avoid the moderation.
There are probably the options
- I am paranoid delusional
- I am lying
- I am having a psychotic episode
- I am high on drugs
There is also the distinct possibility I am telling the truth and I was just dam unlucky to get moderated.
Yes, all those are possible options. The one that isn't is "I'm telling the truth".
What? you wanted me to say "nooo, you're totally right!".
No, patently (twice now) you aren't.
Here, for example is a little fact you probably aren't aware of because you'd prefer to be persecuted for your beliefs:
post two links and you get "this comment is awaiting moderation".
Do you know how I know?
It happens to EVERYONE.
But, no, you're probably being singled out because, well, who the hell knows. It's YOUR paranoid delusion. Enjoy it.
@Ragtag Media #3
You know who else was obsessed with microwaves? Hitler! The Nazis were was also a big fans of ovens. It was Hitler's scientists that combined the two ideas to "invent the microwave oven.
You guys let a contentious thread get to 10 posts before invoking Hitler?!? Pick up your game. That is just embarrassing.
Ethan - how about if you can't stomach the anomalies you just don't talk about. What you are doing in your piece, that you cannot even bring yourself to speak directly about, is you are misleading and you make yourself party to a widespread behaviour in doing so.
Why not just leave it, so the scientist that might have sat down and started to see what it meant doesn't decide to do something because he read your blog.
"how about if you can’t stomach the anomalies you just don’t talk about. "
Throw up.
"you are misleading "
Yeah, just like that,
So you don't like the anomalies. Fine. I refer you to Feynman:
you don't have the right to be dishonest. Too many people are dishonest...they rationalise it's for a just cause or whatever. But who are you to say? Who are you to misinform me?
Who said I didn't like the anomalies?
You asked "if you don't like...".
I answered. If you didn't want it answered, don't ask a question.
Easy enough.
Only you are misinforming you.
"Who said I didn’t like the anomalies?
You asked “if you don’t like…”.
I answered. If you didn’t want it answered, don’t ask a question.
Easy enough.
Only you are misinforming you."
Do you think you're being witty? What you actually are being is tedious.
No, I didn't think I was being witty. Did you? You didn't make any sense whatsoever with ANY of your posts and this one is merely bitch whining.
You come here and claim you're being misinformed by Ethan. Then you claim you're being misinformed by me. And now you're claiming I'm being tedious?
Don't you know what tedious means? Saying the same tired old shit again and again.
Why don't you fuck off if you think you're being misinformed and unappreciated.
Tell you what, I will if you will. I mean you're here all the time. You either are Ethan or you are his unemployed lover scratching a living by antagonising commenters with honest POVs.
l
Tell you what, you do it.
You're in the fast belief that you're being misled. Continuing here is contraindicated if it's true, and demonstrably counterproductive if you're not.
"Tell you what, you do it.
You’re in the fast belief that you’re being misled. Continuing here is contraindicated if it’s true, and demonstrably counterproductive if you’re not"
you little mini-me
nope, I'm not your clone. sorry. your parents misled you.
just like everyone else in your life.
maybe you need to reexamine where the problem lies...
I'd like to do this interactive banter with you for a while longer, because I know that I'm mildly entertained whereas you are seething.
However I probably have to go do some grown up things.
But you're not off the hook yet mini-me
sorry, you don't seem to be here for science, only rhetoric. since I skipped what you wrote after noting who it was from, this appears to have been a waste of your time. do with this information what you like.
A question I've had for some time. Whether in classes or in consulting, we stress that having data that is perfectly normally distributed never happens, since normal distributions are idealized models that make decision making especially easy. In practice, data sets resemble normal distributions in a region loosely described as "the middle", but the differences occur in the tails, where we are usually interested. This is part of the reason robust methods are so useful.
So, for these comments (from two different areas of the Medium article):
Both are clearly based on the assumption of normally distributed data. Is that simply an assumption which is made by default, or is there some bit of mathematics that has been done that indicates the data are close enough in resemblance to what you'd expect from normality to be comfortable with? (If there's a reference that discusses feel free to post that rather than going through the details here.)
dean, yes, there ARE statistics that try to use a different model, and bayesian tries to do without any model at all of the underlying "reality" of their statistical outputs.
I've NEVER used gaussian probabilities for the tosses of N coins where N is a number less than about 100. The more accurate Binomial theory, known by every school child over 13 I'd hope, is more correct and easily handles this case. In a case of 10 million cases, the difference between binomial and gaussian (or poisson) are miniscule compared to any realistic actual error that they can be approximated to gaussian, and there are many things that have already been worked out for the latter so using it doesn't require redoing anything.
When your process is much less well known than a two sided coin, a large dataset will also be approximately the same as gaussian for any like event, though you may have to convert the supposed reality for a simplified one that can be mapped to gaussian. With the shortening of the way noted above for 10 million coin tosses.
A level (post 16 year old) mathematics should give you a starter on this, unless "kids today"...
A first year undergrad work on statistics should cover it well too. After that level, you're talking rather specialist. Ask a specialist.
"Ask a specialist". I am a statistician, but not a physicist, and I asked the questions I did because I see no reasons in that article to justify the assumption of normality. Since the predictions made work, there must be some available.
One of the problems with bayesian work is that it is difficult to avoid many of the issues that arise from non-bayesian analysis. There is some good work being done on making bayesian methods more robust, but it is still in early stages.
"When your process is much less well known than a two sided coin, a large dataset will also be approximately the same as gaussian for any like event"
Not always true - even for these large data sets the tails tend to be heavier than we expect from normal data, and it is the tails where the most interesting information sits. There are situations where, regardless of the sample size, normality is never approached - mathematically the average of the distributions gives the original distribution. More importantly, normal distributions do combine nicely when variances are close to equal, but the multipole graphic (just prior to Ethan's reference to cosmic variance, to anchor my reference point) indicates to me that the data averaged doesn't have that.
And in the one case he mentions, only 5 observations are combined - far too small a sample to be certain of the normality assumption based on the information in that article. Those are the reasons I asked my question.
Well, if you're talking about the medium.com article rather than the stub here, I can't help because I don't go there.
Ask there. Everyone there has at least had the opportunity to read what you're querying.
You need to wait for any different answers. Realise your approach to being answered may have put many others off the idea.
Look over this site for future reference:
http://www.catb.org/esr/faqs/smart-questions.html