"Which is more likely? That the universe was designed just for us, or that we see the universe as having been designed just for us?" -Michael Shermer
One of the problems with the Standard Model of particle physics is known as the hierarchy problem. If you were to calculate the masses of the fundamental particles from first principles, you’d get something on the order of the only natural mass scale that nature provides: the Planck mass, at 10^19 GeV or so. With a lower mass value, the Higgs can account for the smallness of the other masses, but at just 125 GeV, there needs to be some explanation for the Higgs.
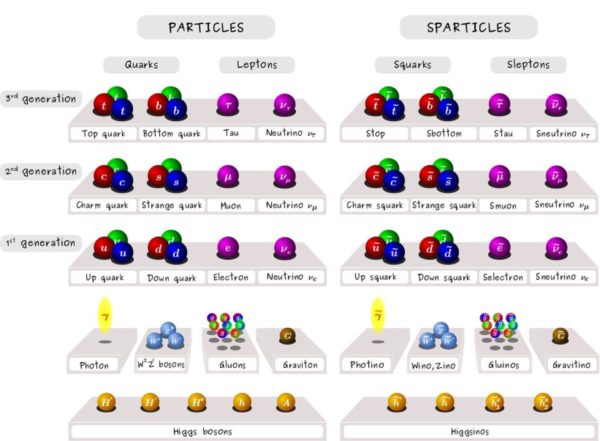The Standard Model particles and their supersymmetric counterparts. Exactly 50% of these particles have been discovered, and 50% have never showed a trace that they exist. Image credit: Claire David, of http://davidc.web.cern.ch/davidc/index.php?id=research.
Physicists were expecting to find some clues to this at the LHC. Perhaps it would have been supersymmetry, whose particle/superparticle symmetry would have protected the Higgs mass down to a low energy scale. Perhaps it could’ve been extra dimensions, technicolor, or any type of new physics. But the LHC found nothing else at all; nothing beyond the Standard Model. What, then does that mean?
The particle tracks emanating from a high energy collision at the LHC in 2014. Image credit: Wikimedia Commons user Pcharito, under a c.c.a.-by-s.a.-3.0 license.
Does it mean that “naturalness” isn’t as natural as we think? That there’s something undiscovered just beyond our reach? Or that we need to appeal to the multiverse to avoid catastrophe? Sabine Hossenfelder has a careful consideration of what the answer might be... and isn’t!
- Log in to post comments
More like this
How can we scientifically prove that we live in a world with no additional dimensions? Could it be that we really live in 11 large dimensions world, but "see" only 4 of them as a limitation of our experiments equipment?
@1: I believe the answer to your question is that we would feel the effects, the same way an ant on a 2-D piece of paper could measure if the paper was warped in the third dimension (and maybe succumb to gravity if you turned the paper upside down). IIRC the string theory concept of large numbers of dimensions includes the caveat that the extra dimensions are very compact and largely empty.
Isn't "a world with no additional dimensions" the null hypothesis? It seems like science would have to demonstrate there are additional dimensions, not that there aren't.
The standard model requires higher dimensions, but rolled up to work, therefore if the standard model is correct, we have extra dimensions.
Yes, that's begging the question of the standard model being right, but we have other theories working out and they're not doing as well explaining the stuff we can currently see or test.
Wow,
I may be misunderstanding things here, but I thought that the Standard Model did NOT require any higher dimensions, but rather it was string theory that required them. Am I wrong about this?
I'll check, but my memory was that symmetry was the reason for the higher dimensions (string theory liking them too). However, it may be that string theory wanted them first, then symmetry in the standard model posited that there would be extra dimensions too, and that this would change things like symmetry and the number of particles.
A lot of models have consequential issues like this: it's how you test whether your theories are right, and which bits.
Of course, i may be getting this wrong. Unfortunately, not available for a week, so by the time I work out what I meant, someone may already have clarified it.
@greg #1: I misread your question the first time...
You asked, "Could it be that we really live in 11 large dimensions world, but “see” only 4 of them as a limitation of our experiments equipment?"
No, and the reason is actually amazingly simple! The apparent intensity of a light source drops like the reciprocal of the distance squared (1/r^2). Similarly, the force of gravity falls of like 1/r^2, as does the electrostatic force due to a point (or spherical) charge.
All of those cases are only true for the case of three spatial dimensions, and it is simply because of the relationship between surface and volume. In two dimensions, the relationships would be 1/r. In four spatial dimensions, the relationships would all be 1/r^3.
In fact, it is the precise measurement of gravity which has allowed us to set limits on _small_ extra dimensions! In bulk, we have tested Newton's 1/r^2 relation very precisely (the Eotvos experiment). Similar measurements have tested that relationship down to distances of less than a millimeter, and we haven't yet found a deviation from 1/r^2.