Sunday Chess Problem

I guess it's been clear that I haven't been in a blogging mood lately. There's plenty of fodder out there, but somehow every time I sit down to write about it I suddenly remember I had something else to do. But that doesn't mean that fans of Sunday Chess Problem should suffer! So this week I have something exotic for you. For the first time in this series we shall consider a retrograde analysis chess problem, or retro for short. It was composed by Raymond Smullyan. Have a look at this position:

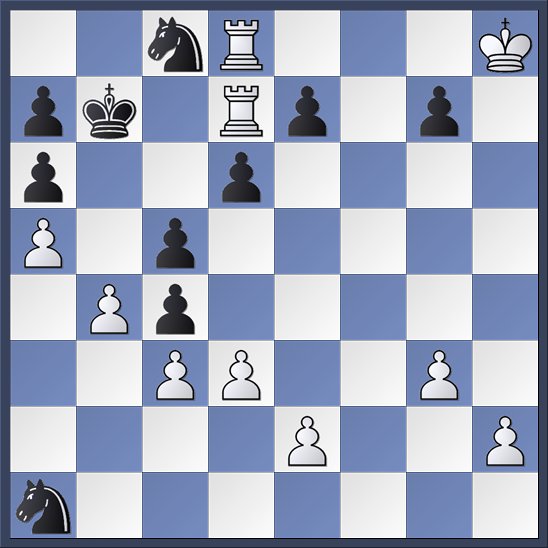
We are to imagine that this position occurred in the course of an actual game of chess. You should assume that the players always made legal moves, but they did not always make sensible moves.

Now direct your attention to the square h4. There is supposed to be a piece standing on that square, but the family dog came in and knocked it off the board. The piece is now hopelessly mixed in with the other captured pieces. Your task is to determine which piece is supposed to be standing on h4. I want both the color and the denomination.

Quite a challenge! If you have never seen such things before, you might be uncertain how to begin. The key is that this position arose in the course of a legal game of chess. That suggests a way forward. You see, black is now in check. That means white has just moved. What move did he make to give this check? Since the rook on d7 has no legal last move, we quickly realize there is only one possibility: A white pawn on c7 just captured a black piece on d8 and promoted to a rook. Why a rook and not a queen? Who cares! We care about legal moves, not sensible moves.

We conclude that just prior to white's last move there was a black piece standing on d8. Which piece was it? If it was a queen, then the position a move ago must have been this:

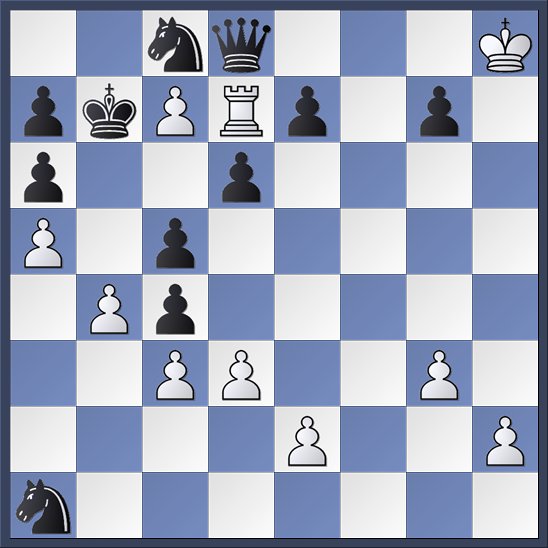
Do you see why this is impossible? Now it is white who is in check, but black has no possible last move to deliver this check. So this is impossible. It is likewise impossible that white just captured a rook.

If white just captured a knight then a move ago the position looked like this:

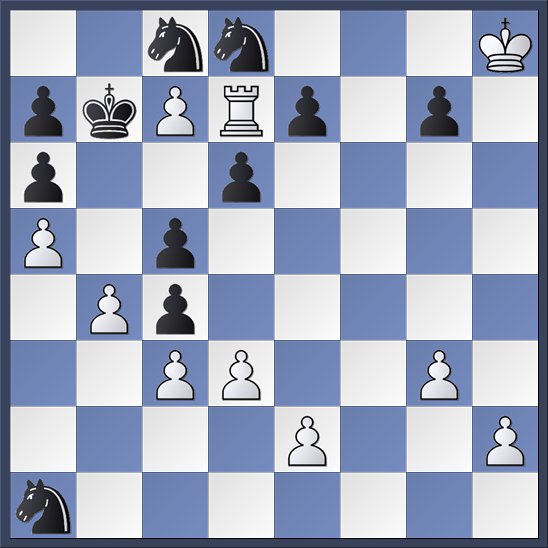
Surely it jumps out at you that there are three black knights on the board. That means that, in this scenario, the one missing black pawn must have promoted to a knight sometime earlier in the game.

A final possibility is that white captured a black bishop:

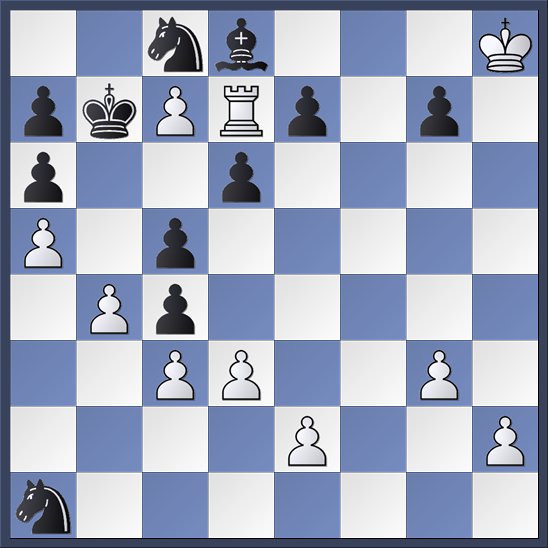
This is a very interesting possibility. Black's dark-squared bishop started the game on f8. But the pawns on e7 and g7 have plainly not moved during the game. This means that the bishop could never have escaped from f8, and must simply have been captured there. Which means that any dark-squared bishop standing on d8 must have been a promoted pawn.

We have thus shown that the one missing black pawn promoted to either a bishop or a knight. We don't know which, but that is OK.

We now have enough information to show that the missing piece is white. For suppose otherwise. What black piece could possibly be standing on h4? Certainly not a rook or a queen, as then both kings would be in check at the same time, which is not possible. It is not the missing black pawn, since that pawn promoted. It cannot be the piece to which the pawn promoted, since that piece was captured on d8. And it cannot be a knight or a bishop, since that would entail a second pawn promotion, but black is only missing one pawn. That exhausts the possibilities.

So far, so good! But how can we determine which white piece it is? This is where things get tricky. The key is to ask yourself about the promoting black pawn. It must have started its life on h7. This is clear from a consideration of black's pawn structure. The pawn on a6 clearly started on b7, while the pawn on c4 could only have started on f7.

Now, the pawn on h7 could not have plowed straight down the h-file, since the white pawn on h2 is in its way. So it must have made at least one capture. Can we be more precise? Indeed we can! You see, since white has ten pieces on the board he is missing six. One of those is the pawn that promoted. That leaves five pieces available for capture. The black pawn on a6 made one capture, while the black pawn on c4 made three. That only leaves one piece available for the h-pawn. We conclude that the black pawn on h7 made one capture over to the g-file and then promoted on g1.

Let's keep going. On what square did the black pawn make its capture? The obvious answer is that the pawn marched down to h3, and then captured on g2, thereby getting around the white pawn on g3. This turns out to be correct, but we need to eliminate another possibility. It could be that the white pawn on g3 began the game on f2. If that is true, then the black pawn could have made its capture anywhere on the g-file, promoted on g1, and then at some later time the white pawn on f2 captured over to g3.

I claim, though, that this is impossible. Notice that black also has ten pieces on the board, leaving six available for capture. Suppose that the pawn now on g3 started on f2. Then it made one capture. Also, the white pawn that just promoted must have started on g2. It made four captures to get to c7, and then a fifth capture when it promoted. That makes a total of six captures, perfectly balanced by black's six missing pieces. It seems that the account balances perfectly, until you remember that the black dark-squared bishop was captured at home on f8! So really there were only five black pieces available for capture, which means this scenario entails one too many.

It follows that the black h-pawn really did make its capture on g2 after all. Do you see why this is relevant?

Five of the six missing white pieces were captured by pawns during the game, while the sixth is currently standing on h4. We actually know the precise squares on which the five pieces were captured: a6, e6, d5, c4, g2. What do those squares have in common? They are all white squares. All of the missing white pieces were captured on white squares.

But there is exactly one white piece that simply cannot be captured on a white square: the dark-squared bishop. That makes the conclusion inescapable: the missing piece is a white bishop.

What do you think?